Help | Advanced Search

Mathematics > General Mathematics
Title: proof of the riemann hypothesis.
Abstract: The Riemann hypothesis, stating that the real part of all non-trivial zero points fo the zeta function must be $\frac{1}{2}$, is one of the most important unproven hypothesises in number theory. In this paper we will proof the Riemann hypothesis by using the integral representation $\zeta(s)=\frac{s}{s-1}-s\int_{1}^{\infty}\frac{x-\lfloor x\rfloor}{x^{s+1}}\,\text{d}x$ and solving the integral for the real part of the zeta function.
Submission history
Access paper:.
- Other Formats
References & Citations
- Google Scholar
- Semantic Scholar
BibTeX formatted citation
Bibliographic and Citation Tools
Code, data and media associated with this article, recommenders and search tools.
- Institution
arXivLabs: experimental projects with community collaborators
arXivLabs is a framework that allows collaborators to develop and share new arXiv features directly on our website.
Both individuals and organizations that work with arXivLabs have embraced and accepted our values of openness, community, excellence, and user data privacy. arXiv is committed to these values and only works with partners that adhere to them.
Have an idea for a project that will add value for arXiv's community? Learn more about arXivLabs .
share this!
January 19, 2022
Quantum zeta epiphany: Physicist finds a new approach to a $1 million mathematical enigma
by University of California - Santa Barbara
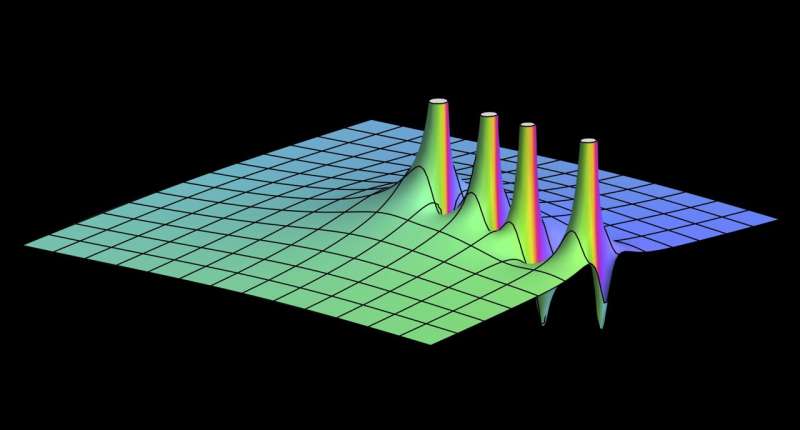
Numbers like π, e and φ often turn up in unexpected places in science and mathematics. Pascal's triangle and the Fibonacci sequence also seem inexplicably widespread in nature. Then there's the Riemann zeta function, a deceptively straightforward function that has perplexed mathematicians since the 19th century. The most famous quandary, the Riemann hypothesis, is perhaps the greatest unsolved question in mathematics, with the Clay Mathematics Institute offering a $1 million prize for a correct proof.
UC Santa Barbara physicist Grant Remmen believes he has a new approach for exploring the quirks of the zeta function. He has found an analog that translates many of the function's important properties into quantum field theory . This means that researchers can now leverage the tools from this field of physics to investigate the enigmatic and oddly ubiquitous zeta function. His work could even lead to a proof of the Riemann hypothesis. Remmen lays out his approach in the journal Physical Review Letters .
"The Riemann zeta function is this famous and mysterious mathematical function that comes up in number theory all over the place," said Remmen, a postdoctoral scholar at UCSB's Kavli Institute for Theoretical Physics. "It's been studied for over 150 years."
An outside perspective
Remmen generally doesn't work on cracking the biggest questions in mathematics. He's usually preoccupied chipping away at the biggest questions in physics. As the fundamental physics fellow at UC Santa Barbara, he normally devotes his attention to topics like particle physics , quantum gravity , string theory and black holes. "In modern high-energy theory, the physics of the largest scales and smallest scales both hold the deepest mysteries," he remarked.
One of his specialties is quantum field theory, which he describes as a "triumph of 20 th century physics." Most people have heard of quantum mechanics (subatomic particles, uncertainty, etc.) and special relativity (time dilation, E=mc 2 , and so forth). "But with quantum field theory, physicists figured out how to combine special relativity and quantum mechanics into a description of how particles moving at or near the speed of light behave," he explained.
Quantum field theory is not exactly a single theory. It's more like a collection of tools that scientists can use to describe any set of particle interactions.
Remmen realized one of the concepts therein shares many characteristics with the Riemann zeta function. It's called a scattering amplitude , and it encodes the quantum mechanical probability that particles will interact with each other. He was intrigued.
Scattering amplitudes often work well with momenta that are complex numbers. These numbers consist of a real part and an imaginary part—a multiple of √-1, which mathematicians call i . Scattering amplitudes have nice properties in the complex plane. For one, they're analytic (can be expressed as a series) around every point except a select set of poles, which all lie along a line.
"That seemed similar to what's going on with the Riemann zeta function's zeros, which all seem to lie on a line," said Remmen. "And so I thought about how to determine whether this apparent similarity was something real."
The scattering amplitude poles correspond to particle production, where a physical event happens that generates a particle with a momentum. The value of each pole corresponds with the mass of the particle that's created. So it was a matter of finding a function that behaves like a scattering amplitude and whose poles correspond to the non-trivial zeros of the zeta function.
With pen, paper and a computer to check his results, Remmen set to work devising a function that had all the relevant properties. "I had had the idea of connecting the Riemann zeta function to amplitudes in the back of my mind for a couple years," he said. "Once I set out to find such a function, it took me about a week to construct it, and fully exploring its properties and writing the paper took a couple months."
Discover the latest in science, tech, and space with over 100,000 subscribers who rely on Phys.org for daily insights. Sign up for our free newsletter and get updates on breakthroughs, innovations, and research that matter— daily or weekly .
Deceptively simple
At its core, the zeta function generalizes the harmonic series:
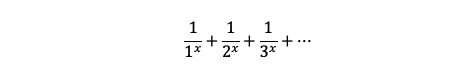
This series blows up to infinity when x ≤ 1, but it converges to an actual number for every x > 1.
In 1859 Bernhard Riemann decided to consider what would happen when x is a complex number. The function, now bearing the name Riemann zeta, takes in one complex number and spits out another.
Riemann also decided to extend the zeta function to numbers where the real component was not greater than 1 by defining it in two parts: the familiar definition holds in places where the function behaves, and another, implicit definition covers the places where it would normally blow up to infinity.
Thanks to a theorem in complex analysis, mathematicians know there is only one formulation for this new area that smoothly preserves the properties of the original function. Unfortunately, no one has been able to represent it in a form with finitely many terms, which is part of the mystery surrounding this function.
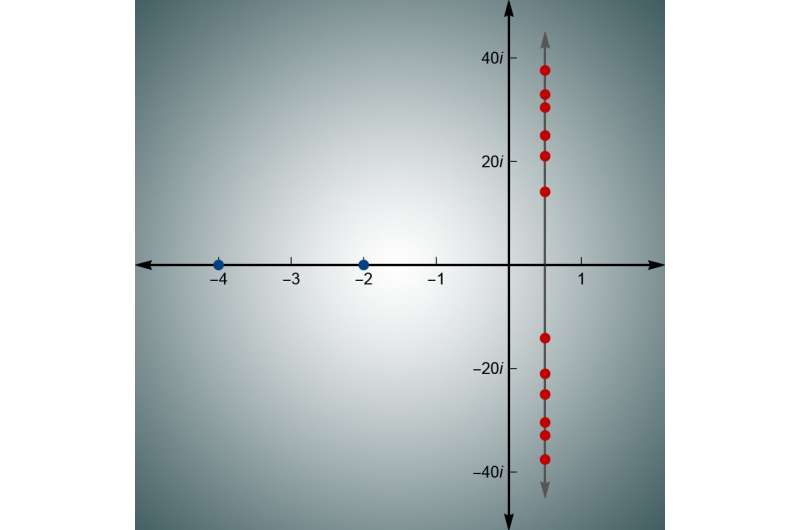
Given the function's simplicity, it should have some nice features. "And yet, those properties end up being fiendishly complicated to understand," Remmen said. For example, take the inputs where the function equals zero. All the negative even numbers are mapped to zero, though this is apparent—or "trivial" as mathematicians say—when the zeta function is written in certain forms. What has perplexed mathematicians is that all of the other, non-trivial zeros appear to lie along a line: Each of them has a real component of ½.
Riemann hypothesized that this pattern holds for all of these non-trivial zeros, and the trend has been confirmed for the first few trillion of them. That said, there are conjectures that work for trillions of examples and then fail at extremely large numbers. So mathematicians can't be certain the hypothesis is true until it's proven.
But if it is true, the Riemann hypothesis has far-reaching implications. "For various reasons it crops up all over the place in fundamental questions in mathematics," Remmen said. Postulates in fields as distinct as computation theory, abstract algebra and number theory hinge on the hypothesis holding true. For instance, proving it would provide an accurate account of the distribution of prime numbers.
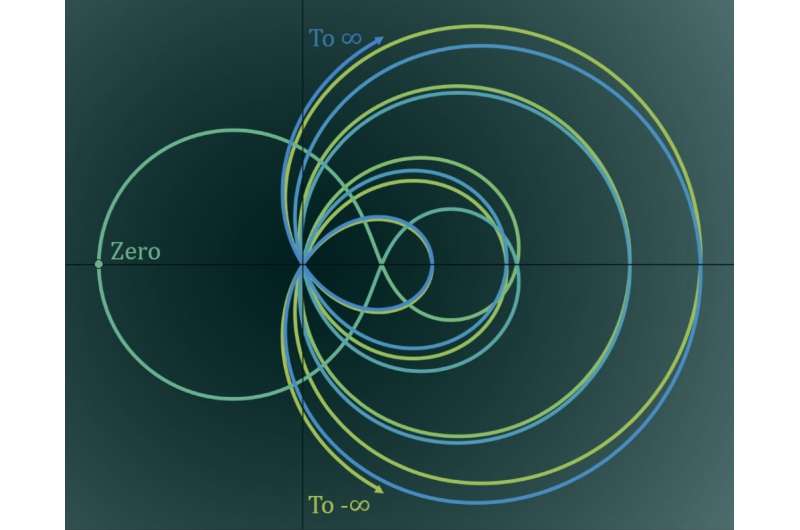
A physical analog
The scattering amplitude that Remmen found describes two massless particles interacting by exchanging an infinite set of massive particles, one at a time. The function has a pole—a point where it cannot be expressed as a series—corresponding to the mass of each intermediate particle. Together, the infinite poles line up with the non-trivial zeros of the Riemann zeta function.
What Remmen constructed is the leading component of the interaction. There are infinitely more that each account for smaller and smaller aspects of the interaction, describing processes involving the exchange of multiple massive particles at once. These "loop-level amplitudes" would be the subject of future work.
The Riemann hypothesis posits that the zeta function's non-trivial zeros all have a real component of ½. Translating this into Remmen's model: All of the amplitude's poles are real numbers. This means that if someone can prove that his function describes a consistent quantum field theory—namely, one where masses are real numbers, not imaginary—then the Riemann hypothesis will be proven.
This formulation brings the Riemann hypothesis into yet another field of science and mathematics, one with powerful tools to offer mathematicians. "Not only is there this relation to the Riemann hypothesis, but there's a whole list of other attributes of the Riemann zeta function that correspond to something physical in the scattering amplitude," Remmen said. For instance, he has already discovered unintuitive mathematical identities related to the zeta function using methods from physics.
Remmen's work follows a tradition of researchers looking to physics to shed light on mathematical quandaries. For instance, physicist Gabriele Veneziano asked a similar question in 1968: whether the Euler beta function could be interpreted as a scattering amplitude. "Indeed it can," Remmen remarked, "and the amplitude that Veneziano constructed was one of the first string theory amplitudes."
Remmen hopes to leverage this amplitude to learn more about the zeta function. "The fact that there are all these analogs means that there's something going on here," he said.
And the approach sets up a path to possibly proving the centuries-old hypothesis. "The innovations necessary to prove that this amplitude does come from a legitimate quantum field theory would, automatically, give you the tools that you need to fully understand the zeta function," Remmen said. "And it would probably give you more as well."
Journal information: Physical Review Letters
Provided by University of California - Santa Barbara
Explore further
Feedback to editors

Earth bids farewell to its temporary 'mini moon' that is possibly a chunk of our actual moon
23 minutes ago

Genetic evidence points to distinct Viking settlers of the Faroe Islands and Iceland
5 hours ago

Wildlife monitoring technologies used to intimidate and spy on women, study finds
10 hours ago

Man scouring Google Earth found a mysterious scar in the Australian outback. Now we know what caused it
14 hours ago

Slender-billed Curlew may be extinct, marking the disappearance of a third bird species from the Western Palaearctic
15 hours ago

WEAVE spectrograph uncovers dual nature of galaxy shock
17 hours ago

Scientists seek miracle pill to stop methane cow burps
23 hours ago

Sustainable method can electrosynthesize important chemical for synthetic rubber production
Nov 23, 2024

Healthy elbow room: Social distancing in Neolithic mega-settlements

First successful test of wild minke whales reveals they have ultrasonic hearing
Relevant physicsforums posts, how do i keep my room cool when my housemate wants the heating on.
Nov 16, 2024
Liquid Flow Calculation out of a pressurized tank
Nov 15, 2024
Questions About Reciprocal Lattice Edge Length
Nov 13, 2024

Explanations about Kardashev type 1 civilizations
Nov 11, 2024
Phase Transition in a Pure Water System
Nov 3, 2024
Trying to identify a weird metal found on a beach
Oct 30, 2024
More from Other Physics Topics
Related Stories

Locations of Riemann zeros accurately measured
Aug 11, 2021

Has one of math's greatest mysteries, the Riemann hypothesis, finally been solved?
Sep 28, 2018

The Riemann conjecture unveiled by physics
Nov 23, 2021

New insight into proving math's million-dollar problem: the Riemann hypothesis (Update)
Apr 7, 2017

Mathematicians revive abandoned approach to the Riemann Hypothesis
May 21, 2019

Quantum simulator offers faster route for prime factorization
Apr 10, 2018
Recommended for you

Extending classical black hole inequalities into the quantum realm

Accessing the lesser known nucleon: New neutron measurement can help physicists learn about nucleon structure and spin
Nov 22, 2024

Discovery of van Hove singularities could lead to novel materials with desirable quantum properties

Scientists develop novel high-fidelity quantum computing gate

Photon qubits challenge AI, enabling more accurate quantum computing without error-correction techniques
Nov 21, 2024

Using matter waves, scientists unveil novel collective behaviors in quantum optics
Nov 20, 2024
Let us know if there is a problem with our content
Use this form if you have come across a typo, inaccuracy or would like to send an edit request for the content on this page. For general inquiries, please use our contact form . For general feedback, use the public comments section below (please adhere to guidelines ).
Please select the most appropriate category to facilitate processing of your request
Thank you for taking time to provide your feedback to the editors.
Your feedback is important to us. However, we do not guarantee individual replies due to the high volume of messages.
E-mail the story
Your email address is used only to let the recipient know who sent the email. Neither your address nor the recipient's address will be used for any other purpose. The information you enter will appear in your e-mail message and is not retained by Phys.org in any form.
Newsletter sign up
Get weekly and/or daily updates delivered to your inbox. You can unsubscribe at any time and we'll never share your details to third parties.
More information Privacy policy
Donate and enjoy an ad-free experience
We keep our content available to everyone. Consider supporting Science X's mission by getting a premium account.
E-mail newsletter

IMAGES
VIDEO