hypothesis test and level of significance

Quality Improvement
Talk To Minitab

Understanding Hypothesis Tests: Significance Levels (Alpha) and P values in Statistics

Topics: Hypothesis Testing , Statistics

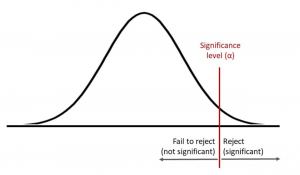
What do significance levels and P values mean in hypothesis tests? What is statistical significance anyway? In this post, I’ll continue to focus on concepts and graphs to help you gain a more intuitive understanding of how hypothesis tests work in statistics.

To bring it to life, I’ll add the significance level and P value to the graph in my previous post in order to perform a graphical version of the 1 sample t-test. It’s easier to understand when you can see what statistical significance truly means!

Here’s where we left off in my last post . We want to determine whether our sample mean (330.6) indicates that this year's average energy cost is significantly different from last year’s average energy cost of $260.

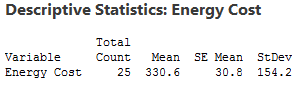
The probability distribution plot above shows the distribution of sample means we’d obtain under the assumption that the null hypothesis is true (population mean = 260) and we repeatedly drew a large number of random samples.

I left you with a question: where do we draw the line for statistical significance on the graph? Now we'll add in the significance level and the P value, which are the decision-making tools we'll need.

We'll use these tools to test the following hypotheses:

Null hypothesis: The population mean equals the hypothesized mean (260).
Alternative hypothesis: The population mean differs from the hypothesized mean (260).

What Is the Significance Level (Alpha)?

The significance level, also denoted as alpha or α, is the probability of rejecting the null hypothesis when it is true. For example, a significance level of 0.05 indicates a 5% risk of concluding that a difference exists when there is no actual difference.

These types of definitions can be hard to understand because of their technical nature. A picture makes the concepts much easier to comprehend!

The significance level determines how far out from the null hypothesis value we'll draw that line on the graph. To graph a significance level of 0.05, we need to shade the 5% of the distribution that is furthest away from the null hypothesis.

Probability plot that shows the critical regions for a significance level of 0.05

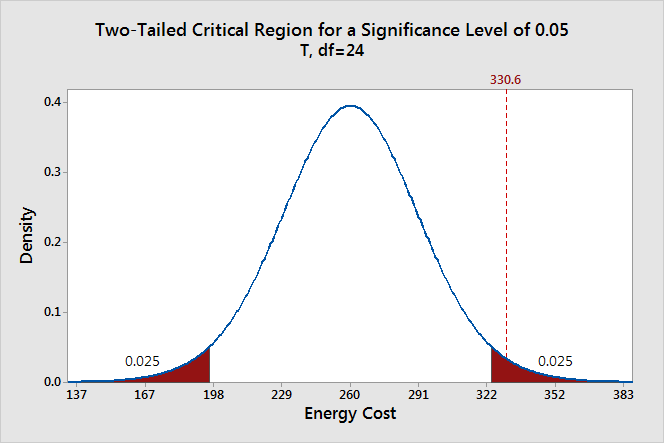
In the graph above, the two shaded areas are equidistant from the null hypothesis value and each area has a probability of 0.025, for a total of 0.05. In statistics, we call these shaded areas the critical region for a two-tailed test. If the population mean is 260, we’d expect to obtain a sample mean that falls in the critical region 5% of the time. The critical region defines how far away our sample statistic must be from the null hypothesis value before we can say it is unusual enough to reject the null hypothesis.

Our sample mean (330.6) falls within the critical region, which indicates it is statistically significant at the 0.05 level.

We can also see if it is statistically significant using the other common significance level of 0.01.

Probability plot that shows the critical regions for a significance level of 0.01

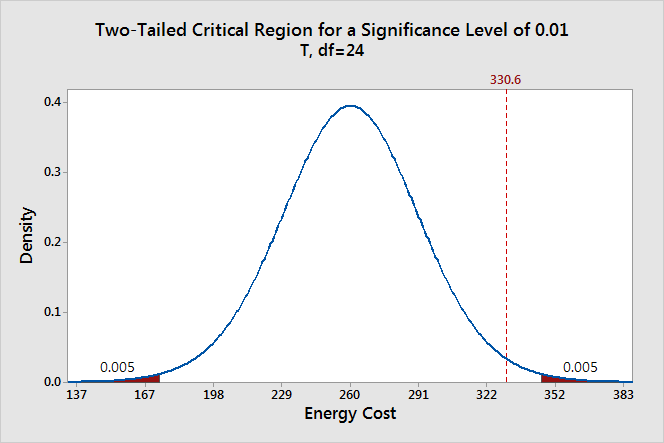
The two shaded areas each have a probability of 0.005, which adds up to a total probability of 0.01. This time our sample mean does not fall within the critical region and we fail to reject the null hypothesis. This comparison shows why you need to choose your significance level before you begin your study. It protects you from choosing a significance level because it conveniently gives you significant results!

Thanks to the graph, we were able to determine that our results are statistically significant at the 0.05 level without using a P value. However, when you use the numeric output produced by statistical software , you’ll need to compare the P value to your significance level to make this determination.

Ready for a demo of Minitab Statistical Software? Just ask!

What Are P values?

P-values are the probability of obtaining an effect at least as extreme as the one in your sample data, assuming the truth of the null hypothesis.

This definition of P values, while technically correct, is a bit convoluted. It’s easier to understand with a graph!

To graph the P value for our example data set, we need to determine the distance between the sample mean and the null hypothesis value (330.6 - 260 = 70.6). Next, we can graph the probability of obtaining a sample mean that is at least as extreme in both tails of the distribution (260 +/- 70.6).

Probability plot that shows the p-value for our sample mean

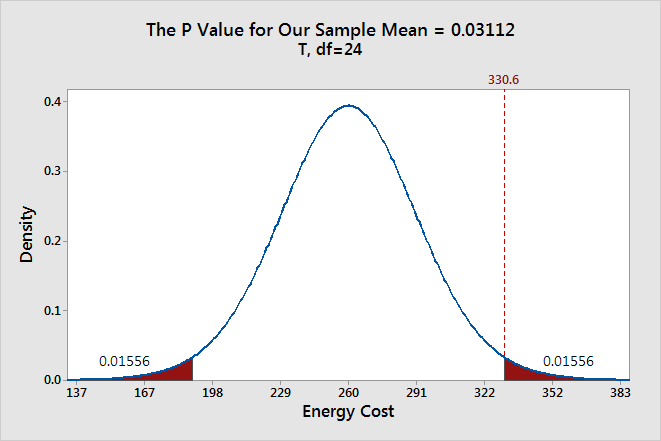
In the graph above, the two shaded areas each have a probability of 0.01556, for a total probability 0.03112. This probability represents the likelihood of obtaining a sample mean that is at least as extreme as our sample mean in both tails of the distribution if the population mean is 260. That’s our P value!

When a P value is less than or equal to the significance level, you reject the null hypothesis. If we take the P value for our example and compare it to the common significance levels, it matches the previous graphical results. The P value of 0.03112 is statistically significant at an alpha level of 0.05, but not at the 0.01 level.

If we stick to a significance level of 0.05, we can conclude that the average energy cost for the population is greater than 260.

A common mistake is to interpret the P-value as the probability that the null hypothesis is true. To understand why this interpretation is incorrect, please read my blog post How to Correctly Interpret P Values .

Discussion about Statistically Significant Results

A hypothesis test evaluates two mutually exclusive statements about a population to determine which statement is best supported by the sample data. A test result is statistically significant when the sample statistic is unusual enough relative to the null hypothesis that we can reject the null hypothesis for the entire population. “Unusual enough” in a hypothesis test is defined by:

The assumption that the null hypothesis is true—the graphs are centered on the null hypothesis value.
The significance level—how far out do we draw the line for the critical region?
Our sample statistic—does it fall in the critical region?

Keep in mind that there is no magic significance level that distinguishes between the studies that have a true effect and those that don’t with 100% accuracy. The common alpha values of 0.05 and 0.01 are simply based on tradition. For a significance level of 0.05, expect to obtain sample means in the critical region 5% of the time when the null hypothesis is true . In these cases, you won’t know that the null hypothesis is true but you’ll reject it because the sample mean falls in the critical region. That’s why the significance level is also referred to as an error rate!

This type of error doesn’t imply that the experimenter did anything wrong or require any other unusual explanation. The graphs show that when the null hypothesis is true, it is possible to obtain these unusual sample means for no reason other than random sampling error. It’s just luck of the draw.

Significance levels and P values are important tools that help you quantify and control this type of error in a hypothesis test. Using these tools to decide when to reject the null hypothesis increases your chance of making the correct decision.

If you like this post, you might want to read the other posts in this series that use the same graphical framework:

Previous: Why We Need to Use Hypothesis Tests
Next: Confidence Intervals and Confidence Levels

If you'd like to see how I made these graphs, please read: How to Create a Graphical Version of the 1-sample t-Test .

Trust Center

Terms of Use
Privacy Policy
Cookies Settings

Warning: The NCBI web site requires JavaScript to function. more...

An official website of the United States government

The .gov means it's official. Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you're on a federal government site.

The site is secure. The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Publications
Account settings
Browse Titles

NCBI Bookshelf. A service of the National Library of Medicine, National Institutes of Health.

StatPearls [Internet]. Treasure Island (FL): StatPearls Publishing; 2024 Jan-.

StatPearls [Internet].

Hypothesis testing, p values, confidence intervals, and significance.

Jacob Shreffler ; Martin R. Huecker .

Affiliations

Last Update: March 13, 2023 .

Definition/Introduction

Medical providers often rely on evidence-based medicine to guide decision-making in practice. Often a research hypothesis is tested with results provided, typically with p values, confidence intervals, or both. Additionally, statistical or research significance is estimated or determined by the investigators. Unfortunately, healthcare providers may have different comfort levels in interpreting these findings, which may affect the adequate application of the data.

Issues of Concern

Without a foundational understanding of hypothesis testing, p values, confidence intervals, and the difference between statistical and clinical significance, it may affect healthcare providers' ability to make clinical decisions without relying purely on the research investigators deemed level of significance. Therefore, an overview of these concepts is provided to allow medical professionals to use their expertise to determine if results are reported sufficiently and if the study outcomes are clinically appropriate to be applied in healthcare practice.

Hypothesis Testing

Investigators conducting studies need research questions and hypotheses to guide analyses. Starting with broad research questions (RQs), investigators then identify a gap in current clinical practice or research. Any research problem or statement is grounded in a better understanding of relationships between two or more variables. For this article, we will use the following research question example:

Research Question: Is Drug 23 an effective treatment for Disease A?

Research questions do not directly imply specific guesses or predictions; we must formulate research hypotheses. A hypothesis is a predetermined declaration regarding the research question in which the investigator(s) makes a precise, educated guess about a study outcome. This is sometimes called the alternative hypothesis and ultimately allows the researcher to take a stance based on experience or insight from medical literature. An example of a hypothesis is below.

Research Hypothesis: Drug 23 will significantly reduce symptoms associated with Disease A compared to Drug 22.

The null hypothesis states that there is no statistical difference between groups based on the stated research hypothesis.

Researchers should be aware of journal recommendations when considering how to report p values, and manuscripts should remain internally consistent.

Regarding p values, as the number of individuals enrolled in a study (the sample size) increases, the likelihood of finding a statistically significant effect increases. With very large sample sizes, the p-value can be very low significant differences in the reduction of symptoms for Disease A between Drug 23 and Drug 22. The null hypothesis is deemed true until a study presents significant data to support rejecting the null hypothesis. Based on the results, the investigators will either reject the null hypothesis (if they found significant differences or associations) or fail to reject the null hypothesis (they could not provide proof that there were significant differences or associations).

To test a hypothesis, researchers obtain data on a representative sample to determine whether to reject or fail to reject a null hypothesis. In most research studies, it is not feasible to obtain data for an entire population. Using a sampling procedure allows for statistical inference, though this involves a certain possibility of error. [1] When determining whether to reject or fail to reject the null hypothesis, mistakes can be made: Type I and Type II errors. Though it is impossible to ensure that these errors have not occurred, researchers should limit the possibilities of these faults. [2]

Significance

Significance is a term to describe the substantive importance of medical research. Statistical significance is the likelihood of results due to chance. [3] Healthcare providers should always delineate statistical significance from clinical significance, a common error when reviewing biomedical research. [4] When conceptualizing findings reported as either significant or not significant, healthcare providers should not simply accept researchers' results or conclusions without considering the clinical significance. Healthcare professionals should consider the clinical importance of findings and understand both p values and confidence intervals so they do not have to rely on the researchers to determine the level of significance. [5] One criterion often used to determine statistical significance is the utilization of p values.

P values are used in research to determine whether the sample estimate is significantly different from a hypothesized value. The p-value is the probability that the observed effect within the study would have occurred by chance if, in reality, there was no true effect. Conventionally, data yielding a p<0.05 or p<0.01 is considered statistically significant. While some have debated that the 0.05 level should be lowered, it is still universally practiced. [6] Hypothesis testing allows us to determine the size of the effect.

An example of findings reported with p values are below:

Statement: Drug 23 reduced patients' symptoms compared to Drug 22. Patients who received Drug 23 (n=100) were 2.1 times less likely than patients who received Drug 22 (n = 100) to experience symptoms of Disease A, p<0.05.

Statement:Individuals who were prescribed Drug 23 experienced fewer symptoms (M = 1.3, SD = 0.7) compared to individuals who were prescribed Drug 22 (M = 5.3, SD = 1.9). This finding was statistically significant, p= 0.02.

For either statement, if the threshold had been set at 0.05, the null hypothesis (that there was no relationship) should be rejected, and we should conclude significant differences. Noticeably, as can be seen in the two statements above, some researchers will report findings with < or > and others will provide an exact p-value (0.000001) but never zero [6] . When examining research, readers should understand how p values are reported. The best practice is to report all p values for all variables within a study design, rather than only providing p values for variables with significant findings. [7] The inclusion of all p values provides evidence for study validity and limits suspicion for selective reporting/data mining.

While researchers have historically used p values, experts who find p values problematic encourage the use of confidence intervals. [8] . P-values alone do not allow us to understand the size or the extent of the differences or associations. [3] In March 2016, the American Statistical Association (ASA) released a statement on p values, noting that scientific decision-making and conclusions should not be based on a fixed p-value threshold (e.g., 0.05). They recommend focusing on the significance of results in the context of study design, quality of measurements, and validity of data. Ultimately, the ASA statement noted that in isolation, a p-value does not provide strong evidence. [9]

When conceptualizing clinical work, healthcare professionals should consider p values with a concurrent appraisal study design validity. For example, a p-value from a double-blinded randomized clinical trial (designed to minimize bias) should be weighted higher than one from a retrospective observational study [7] . The p-value debate has smoldered since the 1950s [10] , and replacement with confidence intervals has been suggested since the 1980s. [11]

Confidence Intervals

A confidence interval provides a range of values within given confidence (e.g., 95%), including the accurate value of the statistical constraint within a targeted population. [12] Most research uses a 95% CI, but investigators can set any level (e.g., 90% CI, 99% CI). [13] A CI provides a range with the lower bound and upper bound limits of a difference or association that would be plausible for a population. [14] Therefore, a CI of 95% indicates that if a study were to be carried out 100 times, the range would contain the true value in 95, [15] confidence intervals provide more evidence regarding the precision of an estimate compared to p-values. [6]

In consideration of the similar research example provided above, one could make the following statement with 95% CI:

Statement: Individuals who were prescribed Drug 23 had no symptoms after three days, which was significantly faster than those prescribed Drug 22; there was a mean difference between the two groups of days to the recovery of 4.2 days (95% CI: 1.9 – 7.8).

It is important to note that the width of the CI is affected by the standard error and the sample size; reducing a study sample number will result in less precision of the CI (increase the width). [14] A larger width indicates a smaller sample size or a larger variability. [16] A researcher would want to increase the precision of the CI. For example, a 95% CI of 1.43 – 1.47 is much more precise than the one provided in the example above. In research and clinical practice, CIs provide valuable information on whether the interval includes or excludes any clinically significant values. [14]

Null values are sometimes used for differences with CI (zero for differential comparisons and 1 for ratios). However, CIs provide more information than that. [15] Consider this example: A hospital implements a new protocol that reduced wait time for patients in the emergency department by an average of 25 minutes (95% CI: -2.5 – 41 minutes). Because the range crosses zero, implementing this protocol in different populations could result in longer wait times; however, the range is much higher on the positive side. Thus, while the p-value used to detect statistical significance for this may result in "not significant" findings, individuals should examine this range, consider the study design, and weigh whether or not it is still worth piloting in their workplace.

Similarly to p-values, 95% CIs cannot control for researchers' errors (e.g., study bias or improper data analysis). [14] In consideration of whether to report p-values or CIs, researchers should examine journal preferences. When in doubt, reporting both may be beneficial. [13] An example is below:

Reporting both: Individuals who were prescribed Drug 23 had no symptoms after three days, which was significantly faster than those prescribed Drug 22, p = 0.009. There was a mean difference between the two groups of days to the recovery of 4.2 days (95% CI: 1.9 – 7.8).

Clinical Significance

Recall that clinical significance and statistical significance are two different concepts. Healthcare providers should remember that a study with statistically significant differences and large sample size may be of no interest to clinicians, whereas a study with smaller sample size and statistically non-significant results could impact clinical practice. [14] Additionally, as previously mentioned, a non-significant finding may reflect the study design itself rather than relationships between variables.

Healthcare providers using evidence-based medicine to inform practice should use clinical judgment to determine the practical importance of studies through careful evaluation of the design, sample size, power, likelihood of type I and type II errors, data analysis, and reporting of statistical findings (p values, 95% CI or both). [4] Interestingly, some experts have called for "statistically significant" or "not significant" to be excluded from work as statistical significance never has and will never be equivalent to clinical significance. [17]

The decision on what is clinically significant can be challenging, depending on the providers' experience and especially the severity of the disease. Providers should use their knowledge and experiences to determine the meaningfulness of study results and make inferences based not only on significant or insignificant results by researchers but through their understanding of study limitations and practical implications.

Nursing, Allied Health, and Interprofessional Team Interventions

All physicians, nurses, pharmacists, and other healthcare professionals should strive to understand the concepts in this chapter. These individuals should maintain the ability to review and incorporate new literature for evidence-based and safe care.

Review Questions
Access free multiple choice questions on this topic.
Comment on this article.

Disclosure: Jacob Shreffler declares no relevant financial relationships with ineligible companies.

Disclosure: Martin Huecker declares no relevant financial relationships with ineligible companies.

This book is distributed under the terms of the Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International (CC BY-NC-ND 4.0) ( http://creativecommons.org/licenses/by-nc-nd/4.0/ ), which permits others to distribute the work, provided that the article is not altered or used commercially. You are not required to obtain permission to distribute this article, provided that you credit the author and journal.

Cite this Page Shreffler J, Huecker MR. Hypothesis Testing, P Values, Confidence Intervals, and Significance. [Updated 2023 Mar 13]. In: StatPearls [Internet]. Treasure Island (FL): StatPearls Publishing; 2024 Jan-.

In this Page

Bulk download.

Bulk download StatPearls data from FTP

Related information

PMC PubMed Central citations
PubMed Links to PubMed

Recent Activity

Hypothesis Testing, P Values, Confidence Intervals, and Significance - StatPearl... Hypothesis Testing, P Values, Confidence Intervals, and Significance - StatPearls

Your browsing activity is empty.

Activity recording is turned off.

Turn recording back on

Connect with NLM

National Library of Medicine 8600 Rockville Pike Bethesda, MD 20894

Web Policies FOIA HHS Vulnerability Disclosure

Help Accessibility Careers

Hypothesis Testing (cont...)

Hypothesis testing, the null and alternative hypothesis.

In order to undertake hypothesis testing you need to express your research hypothesis as a null and alternative hypothesis. The null hypothesis and alternative hypothesis are statements regarding the differences or effects that occur in the population. You will use your sample to test which statement (i.e., the null hypothesis or alternative hypothesis) is most likely (although technically, you test the evidence against the null hypothesis). So, with respect to our teaching example, the null and alternative hypothesis will reflect statements about all statistics students on graduate management courses.

The null hypothesis is essentially the "devil's advocate" position. That is, it assumes that whatever you are trying to prove did not happen ( hint: it usually states that something equals zero). For example, the two different teaching methods did not result in different exam performances (i.e., zero difference). Another example might be that there is no relationship between anxiety and athletic performance (i.e., the slope is zero). The alternative hypothesis states the opposite and is usually the hypothesis you are trying to prove (e.g., the two different teaching methods did result in different exam performances). Initially, you can state these hypotheses in more general terms (e.g., using terms like "effect", "relationship", etc.), as shown below for the teaching methods example:

Null Hypotheses (H ):	Undertaking seminar classes has no effect on students' performance.
Alternative Hypothesis (H ):	Undertaking seminar class has a positive effect on students' performance.

Depending on how you want to "summarize" the exam performances will determine how you might want to write a more specific null and alternative hypothesis. For example, you could compare the mean exam performance of each group (i.e., the "seminar" group and the "lectures-only" group). This is what we will demonstrate here, but other options include comparing the distributions , medians , amongst other things. As such, we can state:

Null Hypotheses (H ):	The mean exam mark for the "seminar" and "lecture-only" teaching methods is the same in the population.
Alternative Hypothesis (H ):	The mean exam mark for the "seminar" and "lecture-only" teaching methods is not the same in the population.

Now that you have identified the null and alternative hypotheses, you need to find evidence and develop a strategy for declaring your "support" for either the null or alternative hypothesis. We can do this using some statistical theory and some arbitrary cut-off points. Both these issues are dealt with next.

Significance levels

The level of statistical significance is often expressed as the so-called p -value . Depending on the statistical test you have chosen, you will calculate a probability (i.e., the p -value) of observing your sample results (or more extreme) given that the null hypothesis is true . Another way of phrasing this is to consider the probability that a difference in a mean score (or other statistic) could have arisen based on the assumption that there really is no difference. Let us consider this statement with respect to our example where we are interested in the difference in mean exam performance between two different teaching methods. If there really is no difference between the two teaching methods in the population (i.e., given that the null hypothesis is true), how likely would it be to see a difference in the mean exam performance between the two teaching methods as large as (or larger than) that which has been observed in your sample?

So, you might get a p -value such as 0.03 (i.e., p = .03). This means that there is a 3% chance of finding a difference as large as (or larger than) the one in your study given that the null hypothesis is true. However, you want to know whether this is "statistically significant". Typically, if there was a 5% or less chance (5 times in 100 or less) that the difference in the mean exam performance between the two teaching methods (or whatever statistic you are using) is as different as observed given the null hypothesis is true, you would reject the null hypothesis and accept the alternative hypothesis. Alternately, if the chance was greater than 5% (5 times in 100 or more), you would fail to reject the null hypothesis and would not accept the alternative hypothesis. As such, in this example where p = .03, we would reject the null hypothesis and accept the alternative hypothesis. We reject it because at a significance level of 0.03 (i.e., less than a 5% chance), the result we obtained could happen too frequently for us to be confident that it was the two teaching methods that had an effect on exam performance.

Whilst there is relatively little justification why a significance level of 0.05 is used rather than 0.01 or 0.10, for example, it is widely used in academic research. However, if you want to be particularly confident in your results, you can set a more stringent level of 0.01 (a 1% chance or less; 1 in 100 chance or less).

One- and two-tailed predictions

When considering whether we reject the null hypothesis and accept the alternative hypothesis, we need to consider the direction of the alternative hypothesis statement. For example, the alternative hypothesis that was stated earlier is:

Alternative Hypothesis (H ):

Undertaking seminar classes has a positive effect on students' performance.

The alternative hypothesis tells us two things. First, what predictions did we make about the effect of the independent variable(s) on the dependent variable(s)? Second, what was the predicted direction of this effect? Let's use our example to highlight these two points.

Sarah predicted that her teaching method (independent variable: teaching method), whereby she not only required her students to attend lectures, but also seminars, would have a positive effect (that is, increased) students' performance (dependent variable: exam marks). If an alternative hypothesis has a direction (and this is how you want to test it), the hypothesis is one-tailed. That is, it predicts direction of the effect. If the alternative hypothesis has stated that the effect was expected to be negative, this is also a one-tailed hypothesis.

Alternatively, a two-tailed prediction means that we do not make a choice over the direction that the effect of the experiment takes. Rather, it simply implies that the effect could be negative or positive. If Sarah had made a two-tailed prediction, the alternative hypothesis might have been:

Alternative Hypothesis (H ):

Undertaking seminar classes has an effect on students' performance.

In other words, we simply take out the word "positive", which implies the direction of our effect. In our example, making a two-tailed prediction may seem strange. After all, it would be logical to expect that "extra" tuition (going to seminar classes as well as lectures) would either have a positive effect on students' performance or no effect at all, but certainly not a negative effect. However, this is just our opinion (and hope) and certainly does not mean that we will get the effect we expect. Generally speaking, making a one-tail prediction (i.e., and testing for it this way) is frowned upon as it usually reflects the hope of a researcher rather than any certainty that it will happen. Notable exceptions to this rule are when there is only one possible way in which a change could occur. This can happen, for example, when biological activity/presence in measured. That is, a protein might be "dormant" and the stimulus you are using can only possibly "wake it up" (i.e., it cannot possibly reduce the activity of a "dormant" protein). In addition, for some statistical tests, one-tailed tests are not possible.

Rejecting or failing to reject the null hypothesis

Let's return finally to the question of whether we reject or fail to reject the null hypothesis.

If our statistical analysis shows that the significance level is below the cut-off value we have set (e.g., either 0.05 or 0.01), we reject the null hypothesis and accept the alternative hypothesis. Alternatively, if the significance level is above the cut-off value, we fail to reject the null hypothesis and cannot accept the alternative hypothesis. You should note that you cannot accept the null hypothesis, but only find evidence against it.

Have a language expert improve your writing

Run a free plagiarism check in 10 minutes, generate accurate citations for free.

Knowledge Base

Hypothesis Testing | A Step-by-Step Guide with Easy Examples

Published on November 8, 2019 by Rebecca Bevans . Revised on June 22, 2023.

Hypothesis testing is a formal procedure for investigating our ideas about the world using statistics . It is most often used by scientists to test specific predictions, called hypotheses, that arise from theories.

There are 5 main steps in hypothesis testing:

State your research hypothesis as a null hypothesis and alternate hypothesis (H o ) and (H a or H 1 ).
Collect data in a way designed to test the hypothesis.
Perform an appropriate statistical test .
Decide whether to reject or fail to reject your null hypothesis.
Present the findings in your results and discussion section.

Though the specific details might vary, the procedure you will use when testing a hypothesis will always follow some version of these steps.

Step 1: state your null and alternate hypothesis, step 2: collect data, step 3: perform a statistical test, step 4: decide whether to reject or fail to reject your null hypothesis, step 5: present your findings, other interesting articles, frequently asked questions about hypothesis testing.

After developing your initial research hypothesis (the prediction that you want to investigate), it is important to restate it as a null (H o ) and alternate (H a ) hypothesis so that you can test it mathematically.

The alternate hypothesis is usually your initial hypothesis that predicts a relationship between variables. The null hypothesis is a prediction of no relationship between the variables you are interested in.

H 0 : Men are, on average, not taller than women. H a : Men are, on average, taller than women.

Here's why students love Scribbr's proofreading services

Discover proofreading & editing

For a statistical test to be valid , it is important to perform sampling and collect data in a way that is designed to test your hypothesis. If your data are not representative, then you cannot make statistical inferences about the population you are interested in.

There are a variety of statistical tests available, but they are all based on the comparison of within-group variance (how spread out the data is within a category) versus between-group variance (how different the categories are from one another).

If the between-group variance is large enough that there is little or no overlap between groups, then your statistical test will reflect that by showing a low p -value . This means it is unlikely that the differences between these groups came about by chance.

Alternatively, if there is high within-group variance and low between-group variance, then your statistical test will reflect that with a high p -value. This means it is likely that any difference you measure between groups is due to chance.

Your choice of statistical test will be based on the type of variables and the level of measurement of your collected data .

an estimate of the difference in average height between the two groups.
a p -value showing how likely you are to see this difference if the null hypothesis of no difference is true.

Based on the outcome of your statistical test, you will have to decide whether to reject or fail to reject your null hypothesis.

In most cases you will use the p -value generated by your statistical test to guide your decision. And in most cases, your predetermined level of significance for rejecting the null hypothesis will be 0.05 – that is, when there is a less than 5% chance that you would see these results if the null hypothesis were true.

In some cases, researchers choose a more conservative level of significance, such as 0.01 (1%). This minimizes the risk of incorrectly rejecting the null hypothesis ( Type I error ).

The results of hypothesis testing will be presented in the results and discussion sections of your research paper , dissertation or thesis .

In the results section you should give a brief summary of the data and a summary of the results of your statistical test (for example, the estimated difference between group means and associated p -value). In the discussion , you can discuss whether your initial hypothesis was supported by your results or not.

In the formal language of hypothesis testing, we talk about rejecting or failing to reject the null hypothesis. You will probably be asked to do this in your statistics assignments.

However, when presenting research results in academic papers we rarely talk this way. Instead, we go back to our alternate hypothesis (in this case, the hypothesis that men are on average taller than women) and state whether the result of our test did or did not support the alternate hypothesis.

If your null hypothesis was rejected, this result is interpreted as “supported the alternate hypothesis.”

These are superficial differences; you can see that they mean the same thing.

You might notice that we don’t say that we reject or fail to reject the alternate hypothesis . This is because hypothesis testing is not designed to prove or disprove anything. It is only designed to test whether a pattern we measure could have arisen spuriously, or by chance.

If we reject the null hypothesis based on our research (i.e., we find that it is unlikely that the pattern arose by chance), then we can say our test lends support to our hypothesis . But if the pattern does not pass our decision rule, meaning that it could have arisen by chance, then we say the test is inconsistent with our hypothesis .

If you want to know more about statistics , methodology , or research bias , make sure to check out some of our other articles with explanations and examples.

Normal distribution
Descriptive statistics
Measures of central tendency
Correlation coefficient

Methodology

Cluster sampling
Stratified sampling
Types of interviews
Cohort study
Thematic analysis

Research bias

Implicit bias
Cognitive bias
Survivorship bias
Availability heuristic
Nonresponse bias
Regression to the mean

Hypothesis testing is a formal procedure for investigating our ideas about the world using statistics. It is used by scientists to test specific predictions, called hypotheses , by calculating how likely it is that a pattern or relationship between variables could have arisen by chance.

A hypothesis states your predictions about what your research will find. It is a tentative answer to your research question that has not yet been tested. For some research projects, you might have to write several hypotheses that address different aspects of your research question.

A hypothesis is not just a guess — it should be based on existing theories and knowledge. It also has to be testable, which means you can support or refute it through scientific research methods (such as experiments, observations and statistical analysis of data).

Null and alternative hypotheses are used in statistical hypothesis testing . The null hypothesis of a test always predicts no effect or no relationship between variables, while the alternative hypothesis states your research prediction of an effect or relationship.

Cite this Scribbr article

If you want to cite this source, you can copy and paste the citation or click the “Cite this Scribbr article” button to automatically add the citation to our free Citation Generator.

Bevans, R. (2023, June 22). Hypothesis Testing | A Step-by-Step Guide with Easy Examples. Scribbr. Retrieved August 21, 2024, from https://www.scribbr.com/statistics/hypothesis-testing/

Is this article helpful?

Rebecca Bevans

Other students also liked, choosing the right statistical test | types & examples, understanding p values | definition and examples, what is your plagiarism score.

Reset password New user? Sign up

Existing user? Log in

Hypothesis Testing

Already have an account? Log in here.

A hypothesis test is a statistical inference method used to test the significance of a proposed (hypothesized) relation between population statistics (parameters) and their corresponding sample estimators . In other words, hypothesis tests are used to determine if there is enough evidence in a sample to prove a hypothesis true for the entire population.

The test considers two hypotheses: the null hypothesis , which is a statement meant to be tested, usually something like "there is no effect" with the intention of proving this false, and the alternate hypothesis , which is the statement meant to stand after the test is performed. The two hypotheses must be mutually exclusive ; moreover, in most applications, the two are complementary (one being the negation of the other). The test works by comparing the $p$-value to the level of significance (a chosen target). If the $p$-value is less than or equal to the level of significance, then the null hypothesis is rejected.

When analyzing data, only samples of a certain size might be manageable as efficient computations. In some situations the error terms follow a continuous or infinite distribution, hence the use of samples to suggest accuracy of the chosen test statistics. The method of hypothesis testing gives an advantage over guessing what distribution or which parameters the data follows.

Definitions and Methodology

Hypothesis test and confidence intervals.

In statistical inference, properties (parameters) of a population are analyzed by sampling data sets. Given assumptions on the distribution, i.e. a statistical model of the data, certain hypotheses can be deduced from the known behavior of the model. These hypotheses must be tested against sampled data from the population.

The null hypothesis $($denoted $H_0)$ is a statement that is assumed to be true. If the null hypothesis is rejected, then there is enough evidence (statistical significance) to accept the alternate hypothesis $($denoted $H_1).$ Before doing any test for significance, both hypotheses must be clearly stated and non-conflictive, i.e. mutually exclusive, statements. Rejecting the null hypothesis, given that it is true, is called a type I error and it is denoted $\alpha$, which is also its probability of occurrence. Failing to reject the null hypothesis, given that it is false, is called a type II error and it is denoted $\beta$, which is also its probability of occurrence. Also, $\alpha$ is known as the significance level , and $1-\beta$ is known as the power of the test. $H_0$ $\textbf{is true}$$\hspace{15mm}$ $H_0$ $\textbf{is false}$ $\textbf{Reject}$ $H_0$$\hspace{10mm}$ Type I error Correct Decision $\textbf{Reject}$ $H_1$ Correct Decision Type II error The test statistic is the standardized value following the sampled data under the assumption that the null hypothesis is true, and a chosen particular test. These tests depend on the statistic to be studied and the assumed distribution it follows, e.g. the population mean following a normal distribution. The $p$-value is the probability of observing an extreme test statistic in the direction of the alternate hypothesis, given that the null hypothesis is true. The critical value is the value of the assumed distribution of the test statistic such that the probability of making a type I error is small.

Methodologies: Given an estimator $\hat \theta$ of a population statistic $\theta$, following a probability distribution $P(T)$, computed from a sample $\mathcal{S},$ and given a significance level $\alpha$ and test statistic $t^*,$ define $H_0$ and $H_1;$ compute the test statistic $t^*.$ $p$-value Approach (most prevalent): Find the $p$-value using $t^*$ (right-tailed). If the $p$-value is at most $\alpha,$ reject $H_0$. Otherwise, reject $H_1$. Critical Value Approach: Find the critical value solving the equation $P(T\geq t_\alpha)=\alpha$ (right-tailed). If $t^*>t_\alpha$, reject $H_0$. Otherwise, reject $H_1$. Note: Failing to reject $H_0$ only means inability to accept $H_1$, and it does not mean to accept $H_0$.

Assume a normally distributed population has recorded cholesterol levels with various statistics computed. From a sample of 100 subjects in the population, the sample mean was 214.12 mg/dL (milligrams per deciliter), with a sample standard deviation of 45.71 mg/dL. Perform a hypothesis test, with significance level 0.05, to test if there is enough evidence to conclude that the population mean is larger than 200 mg/dL. Hypothesis Test We will perform a hypothesis test using the $p$-value approach with significance level $\alpha=0.05:$ Define $H_0$: $\mu=200$. Define $H_1$: $\mu>200$. Since our values are normally distributed, the test statistic is $z^*=\frac{\bar X - \mu_0}{\frac{s}{\sqrt{n}}}=\frac{214.12 - 200}{\frac{45.71}{\sqrt{100}}}\approx 3.09$. Using a standard normal distribution, we find that our $p$-value is approximately $0.001$. Since the $p$-value is at most $\alpha=0.05,$ we reject $H_0$. Therefore, we can conclude that the test shows sufficient evidence to support the claim that $\mu$ is larger than $200$ mg/dL.

If the sample size was smaller, the normal and $t$-distributions behave differently. Also, the question itself must be managed by a double-tail test instead.

Assume a population's cholesterol levels are recorded and various statistics are computed. From a sample of 25 subjects, the sample mean was 214.12 mg/dL (milligrams per deciliter), with a sample standard deviation of 45.71 mg/dL. Perform a hypothesis test, with significance level 0.05, to test if there is enough evidence to conclude that the population mean is not equal to 200 mg/dL. Hypothesis Test We will perform a hypothesis test using the $p$-value approach with significance level $\alpha=0.05$ and the $t$-distribution with 24 degrees of freedom: Define $H_0$: $\mu=200$. Define $H_1$: $\mu\neq 200$. Using the $t$-distribution, the test statistic is $t^*=\frac{\bar X - \mu_0}{\frac{s}{\sqrt{n}}}=\frac{214.12 - 200}{\frac{45.71}{\sqrt{25}}}\approx 1.54$. Using a $t$-distribution with 24 degrees of freedom, we find that our $p$-value is approximately $2(0.068)=0.136$. We have multiplied by two since this is a two-tailed argument, i.e. the mean can be smaller than or larger than. Since the $p$-value is larger than $\alpha=0.05,$ we fail to reject $H_0$. Therefore, the test does not show sufficient evidence to support the claim that $\mu$ is not equal to $200$ mg/dL.

The complement of the rejection on a two-tailed hypothesis test (with significance level $\alpha$) for a population parameter $\theta$ is equivalent to finding a confidence interval $($with confidence level $1-\alpha)$ for the population parameter $\theta$. If the assumption on the parameter $\theta$ falls inside the confidence interval, then the test has failed to reject the null hypothesis $($with $p$-value greater than $\alpha).$ Otherwise, if $\theta$ does not fall in the confidence interval, then the null hypothesis is rejected in favor of the alternate $($with $p$-value at most $\alpha).$

Statistics (Estimation)
Normal Distribution
Correlation
Confidence Intervals

Problem Loading...

Note Loading...

Set Loading...

Prompt Library
DS/AI Trends
Stats Tools
Interview Questions
Generative AI
Machine Learning
Deep Learning

Level of Significance & Hypothesis Testing

In hypothesis testing , the level of significance is a measure of how confident you can be about rejecting the null hypothesis. This blog post will explore what hypothesis testing is and why understanding significance levels are important for your data science projects. In addition, you will also get to test your knowledge of level of significance towards the end of the blog with the help of quiz . These questions can help you test your understanding and prepare for data science / statistics interviews . Before we look into what level of significance is, let’s quickly understand what is hypothesis testing.

Table of Contents

What is Hypothesis testing and how is it related to significance level?

Hypothesis testing can be defined as tests performed to evaluate whether a claim or theory about something is true or otherwise. In order to perform hypothesis tests, the following steps need to be taken:

Hypothesis formulation: Formulate the null and alternate hypothesis
Data collection: Gather the sample of data
Statistical tests: Determine the statistical test and test statistics. The statistical tests can be z-test or t-test depending upon the number of data samples and/or whether the population variance is known otherwise.
Set the level of significance
Calculate the p-value
Draw conclusions: Based on the value of p-value and significance level, reject the null hypothesis or otherwise.

A detailed explanation is provided in one of my related posts titled hypothesis testing explained with examples .

What is the level of significance?

The level of significance is defined as the criteria or threshold value based on which one can reject the null hypothesis or fail to reject the null hypothesis. The level of significance determines whether the outcome of hypothesis testing is statistically significant or otherwise. The significance level is also called as alpha level.

Another way of looking at the level of significance is the value which represents the likelihood of making a type I error . You may recall that Type I error occurs while evaluating hypothesis testing outcomes. If you reject the null hypothesis by mistake, you end up making a Type I error. This scenario is also termed as “false positive”. Take an example of a person alleged with committing a crime. The null hypothesis is that the person is not guilty. Type I error happens when you reject the null hypothesis by mistake. Given the example, a Type I error happens when you reject the null hypothesis that the person is not guilty by mistake. The innocent person is convicted.

The level of significance can take values such as 0.1 , 0.05 , 0.01 . The most common value of the level of significance is 0.05 . The lower the value of significance level, the lesser is the chance of type I error. That would essentially mean that the experiment or hypothesis testing outcome would really need to be highly precise for one to reject the null hypothesis. The likelihood of making a type I error would be very low. However, that does increase the chances of making type II errors as you may make mistakes in failing to reject the null hypothesis. You may want to read more details in relation to type I errors and type II errors in this post – Type I errors and Type II errors in hypothesis testing

The outcome of the hypothesis testing is evaluated with the help of a p-value. If the p-value is less than the level of significance, then the hypothesis testing outcome is statistically significant. On the other hand, if the hypothesis testing outcome is not statistically significant or the p-value is more than the level of significance, then we fail to reject the null hypothesis. The same is represented in the picture below for a right-tailed test. I will be posting details on different types of tail test in future posts.

The picture below represents the concept for two-tailed hypothesis test:

level of significance and two-tailed test

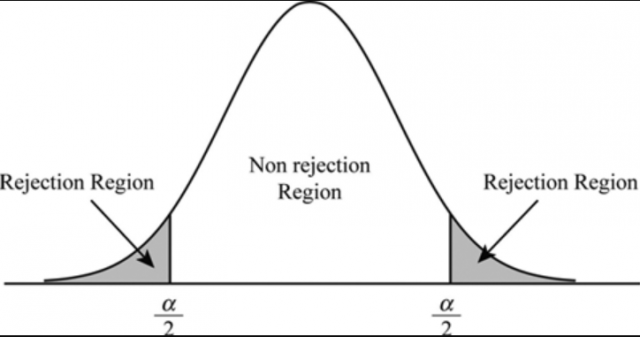
For example: Let’s say that a school principal wants to find out whether extra coaching of 2 hours after school help students do better in their exams. The hypothesis would be as follows:

Null hypothesis : There is no difference between the performance of students even after providing extra coaching of 2 hours after the schools are over.
Alternate hypothesis : Students perform better when they get extra coaching of 2 hours after the schools are over. This hypothesis testing example would require a level of significant value at 0.05 or simply put, it would need to be highly precise that there’s actually a difference between the performance of students based on whether they take extra coaching.

Now, let’s say that we conduct this experiment with 100 students and measure their scores in exams. The test statistics is computed to be z=-0.50 (p-value=0.62). Since the p-value is more than 0.05, we fail to reject the null hypothesis. There is not enough evidence to show that there’s a difference in the performance of students based on whether they get extra coaching.

While performing hypothesis tests or experiments, it is important to keep the level of significance in mind.

Why does one need a level of significance?

In hypothesis tests, if we do not have some sort of threshold by which to determine whether your results are statistically significant enough for you to reject the null hypothesis, then it would be tough for us to determine whether your findings are significant or not. This is why we take into account levels of significance when performing hypothesis tests and experiments.

Since hypothesis testing helps us in making decisions about our data, having a level of significance set up allows one to know what sort of chances their findings might have of actually being due to the null hypothesis. If you set your level of significance at 0.05 for example, it would mean that there’s only a five percent chance that the difference between groups (assuming two groups are tested) is due to random sampling error. So if we found a difference in the performance of students based on whether they take extra coaching, we would need to consider other factors that could have contributed to the difference.

This is why hypothesis testing and level of significance go hand in hand with one another: hypothesis tests help us know whether our data falls within a certain range where it’s statistically significant or not so statistically significant whereas the level of significance tells us how likely is it that our hypothesis testing results are not due to random sampling error.

How is the level of significance used in hypothesis testing?

The level of significance along with the test statistic and p-value formed a key part of hypothesis testing. The value that you derive from hypothesis testing depends on whether or not you accept/reject the null hypothesis, given your findings at each step. Before going into rejection vs non-rejection, let’s understand the terms better.

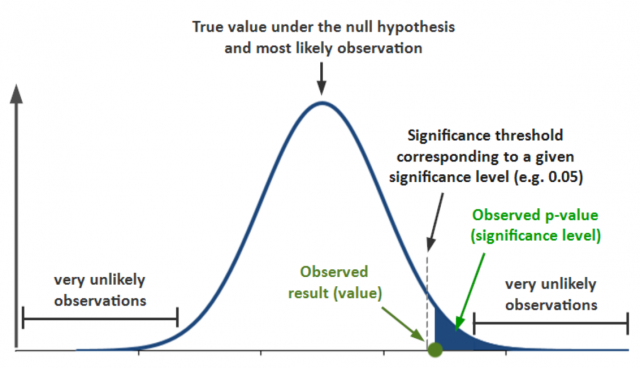
If the test statistic falls within the critical region, you reject the null hypothesis. This means that your findings are statistically significant and support the alternate hypothesis. The value of the p-value determines how likely it is for finding this outcome if, in fact, the null hypothesis were true. If the p-value is less than or equal to the level of significance, you reject the null hypothesis. This means that your hypothesis testing outcome was statistically significant at a certain degree and in favor of the alternate hypothesis.

If on the other hand, the p-value is greater than alpha level or significance level, then you fail to reject the null hypothesis. These findings are not statistically significant enough for one to reject the null hypothesis. The same is represented in the diagram below:

Level of Significance – Quiz / Interview Questions

Here are some practice questions which can help you in testing your questions, and, also prepare for interviews.

#1. The p-value less than the level of significance would mean which of the following?

#2. the statistically significant outcome of hypothesis testing would mean which of the following, #3. which one of the following is considered most popular choice of significance level, #4. which of the following is looks to be inappropriate level of significance, #5. level of significance is also called as ________, #6. the p-value of 0.03 is statistically significant for significance level as 0.01, #7. which of the following will result in greater type i error, #8. which of the following will result in greater type ii error, recent posts.

Self-Supervised Learning: Concepts, Examples - August 20, 2024
MSE vs RMSE vs MAE vs MAPE vs R-Squared: When to Use? - August 18, 2024
K-Fold Cross Validation in Machine Learning – Python Example - August 16, 2024

Oops! Check your answers again. The minimum pass percentage is 70%.

Hypothesis testing is an important statistical concept that helps us determine whether the claim made about anything is true or otherwise. The hypothesis test statistic, level of significance, and p-value all work together to help you make decisions about your data. If our hypothesis tests show enough evidence to reject the null hypothesis, then we know statistically significant findings are at hand. This post gave you ideas for how you can use hypothesis testing in your experiments by understanding what it means when someone rejects or fails to reject the null hypothesis.

Ajitesh Kumar

3 responses.

Well explained with examples and helpful illustration

Thank you for your feedback

Well explained

ChatGPT Prompts (250+)

Generate Design Ideas for App
Expand Feature Set of App
Create a User Journey Map for App
Generate Visual Design Ideas for App
Generate a List of Competitors for App
Self-Supervised Learning: Concepts, Examples
MSE vs RMSE vs MAE vs MAPE vs R-Squared: When to Use?
K-Fold Cross Validation in Machine Learning – Python Example
Gradient Boosting Machines (GBM): Concepts, Examples
Random Forest Classifier – Sklearn Python Example

Data Science / AI Trends

• Prepend any arxiv.org link with talk2 to load the paper into a responsive chat application
• Custom LLM and AI Agents (RAG) On Structured + Unstructured Data - AI Brain For Your Organization
• Guides, papers, lecture, notebooks and resources for prompt engineering
• Common tricks to make LLMs efficient and stable
• Machine learning in finance

Free Online Tools

Create Scatter Plots Online for your Excel Data
Histogram / Frequency Distribution Creation Tool
Online Pie Chart Maker Tool
Z-test vs T-test Decision Tool
Independent samples t-test calculator

Hypothesis Testing and Confidence Intervals

By Jim Frost 20 Comments

Confidence intervals and hypothesis testing are closely related because both methods use the same underlying methodology. Additionally, there is a close connection between significance levels and confidence levels. Indeed, there is such a strong link between them that hypothesis tests and the corresponding confidence intervals always agree about statistical significance.

A confidence interval is calculated from a sample and provides a range of values that likely contains the unknown value of a population parameter . To learn more about confidence intervals in general, how to interpret them, and how to calculate them, read my post about Understanding Confidence Intervals .

In this post, I demonstrate how confidence intervals work using graphs and concepts instead of formulas. In the process, I compare and contrast significance and confidence levels. You’ll learn how confidence intervals are similar to significance levels in hypothesis testing. You can even use confidence intervals to determine statistical significance.

Read the companion post for this one: How Hypothesis Tests Work: Significance Levels (Alpha) and P-values . In that post, I use the same graphical approach to illustrate why we need hypothesis tests, how significance levels and P-values can determine whether a result is statistically significant, and what that actually means.

Significance Level vs. Confidence Level

Let’s delve into how confidence intervals incorporate the margin of error. Like the previous post, I’ll use the same type of sampling distribution that showed us how hypothesis tests work. This sampling distribution is based on the t-distribution , our sample size , and the variability in our sample. Download the CSV data file: FuelsCosts .

There are two critical differences between the sampling distribution graphs for significance levels and confidence intervals–the value that the distribution centers on and the portion we shade.

The significance level chart centers on the null value, and we shade the outside 5% of the distribution.

Conversely, the confidence interval graph centers on the sample mean, and we shade the center 95% of the distribution.

Probability distribution plot that displays 95% confidence interval for our fuel cost dataset.

The shaded range of sample means [267 394] covers 95% of this sampling distribution. This range is the 95% confidence interval for our sample data. We can be 95% confident that the population mean for fuel costs fall between 267 and 394.

Confidence Intervals and the Inherent Uncertainty of Using Sample Data

The graph emphasizes the role of uncertainty around the point estimate . This graph centers on our sample mean. If the population mean equals our sample mean, random samples from this population (N=25) will fall within this range 95% of the time.

We don’t know whether our sample mean is near the population mean. However, we know that the sample mean is an unbiased estimate of the population mean. An unbiased estimate does not tend to be too high or too low. It’s correct on average. Confidence intervals are correct on average because they use sample estimates that are correct on average. Given what we know, the sample mean is the most likely value for the population mean.

Given the sampling distribution, it would not be unusual for other random samples drawn from the same population to have means that fall within the shaded area. In other words, given that we did, in fact, obtain the sample mean of 330.6, it would not be surprising to get other sample means within the shaded range.

If these other sample means would not be unusual, we must conclude that these other values are also plausible candidates for the population mean. There is inherent uncertainty when using sample data to make inferences about the entire population. Confidence intervals help gauge the degree of uncertainty, also known as the margin of error.

Confidence Intervals and Statistical Significance

If you want to determine whether your hypothesis test results are statistically significant, you can use either P-values with significance levels or confidence intervals. These two approaches always agree.

The relationship between the confidence level and the significance level for a hypothesis test is as follows:

Confidence level = 1 – Significance level (alpha)

For example, if your significance level is 0.05, the equivalent confidence level is 95%.

Both of the following conditions represent statistically significant results:

The P-value in a hypothesis test is smaller than the significance level.
The confidence interval excludes the null hypothesis value.

Further, it is always true that when the P-value is less than your significance level, the interval excludes the value of the null hypothesis.

In the fuel cost example, our hypothesis test results are statistically significant because the P-value (0.03112) is less than the significance level (0.05). Likewise, the 95% confidence interval [267 394] excludes the null hypotheses value (260). Using either method, we draw the same conclusion.

Hypothesis Testing and Confidence Intervals Always Agree

The hypothesis testing and confidence interval results always agree. To understand the basis of this agreement, remember how confidence levels and significance levels function:

A confidence level determines the distance between the sample mean and the confidence limits.
A significance level determines the distance between the null hypothesis value and the critical regions.

Both of these concepts specify a distance from the mean to a limit. Surprise! These distances are precisely the same length.

A 1-sample t-test calculates this distance as follows:

The critical t-value * standard error of the mean

Interpreting these statistics goes beyond the scope of this article. But, using this equation, the distance for our fuel cost example is $63.57.

P-value and significance level approach : If the sample mean is more than $63.57 from the null hypothesis mean, the sample mean falls within the critical region, and the difference is statistically significant.

Confidence interval approach : If the null hypothesis mean is more than $63.57 from the sample mean, the interval does not contain this value, and the difference is statistically significant.

Of course, they always agree!

The two approaches always agree as long as the same hypothesis test generates the P-values and confidence intervals and uses equivalent confidence levels and significance levels.

Related posts : Standard Error of the Mean and Critical Values

I Really Like Confidence Intervals!

In statistics, analysts often emphasize using hypothesis tests to determine statistical significance. Unfortunately, a statistically significant effect might not always be practically meaningful. For example, a significant effect can be too small to be important in the real world. Confidence intervals help you navigate this issue!

Similarly, the margin of error in a survey tells you how near you can expect the survey results to be to the correct population value.

Learn more about this distinction in my post about Practical vs. Statistical Significance .

Learn how to use confidence intervals to compare group means !

Finally, learn about bootstrapping in statistics to see an alternative to traditional confidence intervals that do not use probability distributions and test statistics. In that post, I create bootstrapped confidence intervals.

Neyman, J. (1937). Outline of a Theory of Statistical Estimation Based on the Classical Theory of Probability . Philosophical Transactions of the Royal Society A . 236 (767): 333–380.

Reader Interactions

December 7, 2021 at 3:14 pm

I am helping my Physics students use their data to determine whether they can say momentum is conserved. One of the columns in their data chart was change in momentum and ultimately we want this to be 0. They are obviously not getting zero from their data because of outside factors. How can I explain to them that their data supports or does not support conservation of momentum using statistics? They are using a 95% confidence level. Again, we want the change in momentum to be 0. Thank you.

December 9, 2021 at 6:54 pm

I can see several complications with that approach and also my lack of familiarity with the subject area limits what I can say. But here are some considerations.

For starters, I’m unsure whether the outside factors you mention bias the results systematically from zero or just add noise (variability) to the data (but not systematically bias).

If the outside factors bias the results to a non-zero value, then you’d expect the case where larger samples will be more likely to produce confidence intervals that exclude zero. Indeed, only smaller samples sizes might produce CIs that include zero, but that would only be due to the relative lack of precision associated with small sample sizes. In other words, limited data won’t be able to distinguish the sample value from zero even though, given the bias of the outside factors, you’d expect a non-zero value. In other words, if the bias exists, the larger samples will detect the non-zero values correctly while smaller samples might miss it.

If the outside factors don’t bias the results but just add noise, then you’d expect that both small and larger samples will include zero. However, you still have the issue of precision. Smaller samples will include zero because they’re relatively wider intervals. Larger samples should include zero but have narrower intervals. Obviously, you can trust the larger samples more.

In hypothesis testing, when you fail to reject the null, as occurs in the unbiased discussion above, you’re not accepting the null . Click the link to read about that. Failing to reject the null does not mean that the population value equals the hypothesized value (zero in your case). That’s because you can fail to reject the null due to poor quality data (high noise and/or small sample sizes). And you don’t want to draw conclusions based on poor data.

There’s a class of hypothesis testing called equivalence testing that you should use in this case. It flips the null and alternative hypotheses so that the test requires you to collect strong evidence to show that the sample value equals the null value (again, zero in your case). I don’t have a post on that topic (yet), but you can read the Wikipedia article about Equivalence Testing .

I hope that helps!

September 19, 2021 at 5:16 am

Thank you very much. When training a machine learning model using bootstrap, in the end we will have the confidence interval of accuracy. How can I say that this result is statistically significant? Do I have to convert the confidence interval to p-values first and if p-value is less than 0.05, then it is statistically significant?

September 19, 2021 at 3:16 pm

As I mention in this article, you determine significance using a confidence interval by assessing whether it excludes the null hypothesis value. When it excludes the null value, your results are statistically significant.

September 18, 2021 at 12:47 pm

Dear Jim, Thanks for this post. I am new to hypothesis testing and would like to ask you how we know that the null hypotheses value is equal to 260.

Thank you. Kind regards, Loukas

September 19, 2021 at 12:35 am

For this example, the null hypothesis is 260 because that is the value from the previous year and they wanted to compare the current year to the previous year. It’s defined as the previous year value because the goal of the study was to determine whether it has changed since last year.

In general, the null hypothesis will often be a meaningful target value for the study based on their knowledge, such as this case. In other cases, they’ll use a value that represents no effect, such as zero.

I hope that helps clarify it!

February 22, 2021 at 3:49 pm

Hello, Mr. Jim Frost.

Thank you for publishing precise information about statistics, I always read your posts and bought your excellent e-book about regression! I really learn from you.

I got a couple of questions about the confidence level of the confidence intervals. Jacob Cohen, in his article “things I’ve learned (so far)” said that, in his experience, the most useful and informative confidence level is 80%; other authors state that if that level is below 90% it would be very hard to compare across results, as it is uncommon.

My first question is: in exploratory studies, with small samples (for example, N=85), if one wishes to generate correlational hypothesis for future research, would it be better to use a lower confidence level? What is the lowest level you would consider to be acceptable? I ask that because of my own research now, and with a sample size 85 (non-probabilistic sampling) I know all I can do is generate some hypothesis to be explored in the future, so I would like my confidence intervals to be more informative, because I am not looking forward to generalize to the population.

My second question is: could you please provide an example of an appropriate way to describe the information about the confidence interval values/limits, beyond the classic “it contains a difference of 0; it contains a ratio of 1”.

I would really appreciate your answers.

Greetings from Peru!

February 23, 2021 at 4:51 pm

Thanks so much for your kind words and for supporting my regression ebook! I’m glad it’s been helpful! 🙂

On to your questions!

I haven’t read Cohen’s article, so I don’t understand his rationale. However, I’m extremely dubious of using a confidence level as low as 80%. Lowering the confidence level will create a narrower CI, which looks good. However, it comes at the expense of dramatically increasing the likelihood that the CI won’t contain the correct population value! My position is to leave the confidence level at 95%. Or, possibly lower it to 90%. But, I wouldn’t go further. Your CI will be wider, but that’s OK. It’s reflecting the uncertainty that truly exists in your data. That’s important. The problem with lowering the CIs is that it makes your results appear more precise than they actually are.

When I think of exploratory research, I think of studies that are looking at tendencies or trends. Is the overall pattern of results consistent with theoretical expectations and justify further research? At that stage, it shouldn’t be about obtaining statistically significant results–at least not as the primary objective. Additionally, exploratory research can help you derive estimated effect sizes, variability, etc. that you can use for power calculations . A smaller, exploratory study can also help you refine your methodology and not waste your resources by going straight to a larger study that, as a result, might not be as refined as it would without a test run in the smaller study. Consequently, obtaining significant results, or results that look precise when they aren’t, aren’t the top priorities.

I know that lowering the confidence level makes your CI look more information but that is deceptive! I’d resist that temptation. Maybe go down to 90%. Personally, I would not go lower.

As for the interpretation, CIs indicate the likely range that a population parameter is likely to fall within. The parameter can be a mean, effect size, ratio, etc. Often times, you as the researcher are hoping the CI excludes an important value. For example, if the CI is of the effect size, you want the CI to exclude zero (no effect). In that case, you can say that there is unlikely to be no effect in the population (i.e., there probably is a non-zero effect in the population). Additionally, the effect size is likely to be within this range. Other times, you might just want to know the range of values itself. For example, if you have a CI for the mean height of a population, it might be valuable on its own knowing that the population mean height is likely to fall between X and Y. If you have specific example of what the CI assesses, I can give you a more specific interpretation.

Additionally, I cover confidence intervals associated with many different types of hypothesis tests in my Hypothesis Testing ebook . You might consider looking in to that!

July 26, 2020 at 5:45 am

I got a very wide 95% CI of the HR of height in the cox PH model from a very large sample. I already deleted the outliers defined as 1.5 IQR, but it doesn’t work. Do you know how to resolve it?

July 5, 2020 at 6:13 pm

Hello, Jim!

I appreciate the thoughtful and thorough answer you provided. It really helped in crystallizing the topic for me.

If I may ask for a bit more of your time, as long as we are talking about CIs I have another question:

How would you go about constructing a CI for the difference of variances?

I am asking because while creating CIs for the difference of means or proportions is relatively straightforward, I couldn’t find any references for the difference of variances in any of my textbooks (or on the Web for that matter); I did find information regarding CIs for the ratio of variances, but it’s not the same thing.

Could you help me with that?

Thanks a lot!

July 2, 2020 at 6:01 pm

I want to start by thanking you for a great post and an overall great blog! Top notch material.

I have a doubt regarding the difference between confidence intervals for a point estimate and confidence intervals for a hypothesis test.

As I understand, if we are using CIs to test a hypothesis, then our point estimate would be whatever the null hypothesis is; conversely, if we are simply constructing a CI to go along with our point estimate, we’d use the point estimate derived from our sample. Am I correct so far?

The reason I am asking is that because while reading from various sources, I’ve never found a distinction between the two cases, and they seem very different to me.

Bottom line, what I am trying to ask is: assuming the null hypothesis is true, shouldn’t the CI be changed?

Thank you very much for your attention!

July 3, 2020 at 4:02 pm

There’s no difference in the math behind the scenes. The real difference is that when you create a confidence interval in conjunction with a hypothesis test, the software ensures that they’re using consistent methodology. For example, the significance level and confidence level will correspond correctly (i.e., alpha = 0.05 and confidence level = 0.95). Additionally, if you perform a two-tailed test, you will obtain a two-sided CI. On the other hand, if you perform a one-tailed test, you will obtain the appropriate upper or lower bound (i.e., one-sided CIs). The software also ensures any other methodological choices you make will match between the hypothesis test and CI, which ensures the results always agree.

You can perform them separately. However, if you don’t match all the methodology options, the results can differ.

As for your question about assuming the null is true. Keep in mind that hypothesis tests create sampling distributions that center on the null hypothesis value. That’s the assumption that the null is true. However, the sampling distributions for CIs center on the sample estimate. So, yes, CIs change that detail because they don’t assume the null is correct. But that’s always true whether you perform the hypothesis test or not.

Thanks for the great questions!

December 21, 2019 at 6:31 am

Confidence interval has sample static as the most likely value ( value in the center) – and sample distribution assumes the null value to be the most likely value( value in the center). I am a little confused about this. Would be really kind of you if you could show both in the same graph and explain how both are related. How the the distance from the mean to a limit in case of Significance level and CI same?

December 23, 2019 at 3:46 am

That’s a great question. I think part of your confusion is due to terminology.

The sampling distribution of the means centers on the sample mean. This sampling distribution uses your sample mean as its mean and the standard error of the mean as its standard deviation.

The sampling distribution of the test statistic (t) centers on the null hypothesis value (0). This distribution uses zero as its mean and also uses the SEM for its standard deviation.

They’re two different things and center on different points. But, they both incorporate the SEM, which is why they always agree! I do have section in this post about why that distance is always the same. Look for the section titled, “Why They Always Agree.”

November 23, 2019 at 11:31 pm

Hi Jim, I’m the proud owner of 2 of your ebooks. There’s one topic though that keeps puzzling me: If I would take 9 samples of size 15 in order to estimate the population mean, the se of the mean would be substantial larger than if I would take 1 sample of size 135 (divide pop sd by sqrt(15) or sqrt(135) ) whereas the E(x) (or mean of means) would be the same.

Can you please shine a little light on that.

Tx in advance

November 24, 2019 at 3:17 am

Thanks so much for supporting my ebooks. I really appreciate that!! 🙂

So, let’s flip that scenario around. If you know that a single large sample of 135 will produce more precise estimates of the population, why would you collect nine smaller samples? Knowing how statistics works, that’s not a good decision. If you did that in the real world, it would be because there was some practical reason that you could not collect one big example. Further, it would suggest that you had some reason for not being able to combine them later. For example, if you follow the same random sampling procedure on the same population and used all the same methodology and at the same general time, you might feel comfortable combining them together into one larger sample. So, if you couldn’t collect one larger example and you didn’t feel comfortable combining them together, it suggests that you have some reason for doubting that they all measure the same thing for the same population. Maybe you had differences in methodology? Or subjective measurements across different personnel? Or, maybe you collected the samples at different times and you’re worried that the population changed over time?

So, that’s the real world reason for why a researcher would not combine smaller samples into a larger one.

As you can see, the expected value for the population standard deviation is in the numerator (sigma). As the sample size increases, the numerator remains constant (plus or minus random error) because the expected value for the population parameter does not change. Conversely, the square root of the sample size is in the denominator. As the sample size increases, it produces a larger values in the denominator. So, if the expected value of the numerator is constant but the value of the denominator increases with a larger sample size, you expect the SEM to decrease. Smaller SEM’s indicate more precise estimates of the population parameter. For instance, the equations for confidence intervals use the SEM. Hence, for the same population, larger samples tend to produce smaller SEMS, and more precise estimates of the population parameter.

I hope that answers your question!

November 6, 2018 at 10:26 am

first of all: Thanks for your effort and your effective way of explaining!

You say that p-values and C.I.s always agree. I agree.

Why does Tim van der Zee claim the opposite? I’m not enough into statistcs to figure this out.

http://www.timvanderzee.com/not-interpret-confidence-intervals/

Best regards Georg

November 7, 2018 at 9:31 am

I think he is saying that they do agree–just that people often compare the wrong pair of CIs and p-values. I assume you’re referring to the section “What do overlapping intervals (not) mean?” And, he’s correct in what he says. In a 2-sample t-test, it’s not valid to compare the CI for each of the two group means to the test’s p-values because they have different purposes. Consequently, they won’t necessarily agree. However, that’s because you’re comparing results from two different tests/intervals.

On the one hand, you have the CIs for each group. On the other hand, you have the p-value for the difference between the two groups. Those are not the same thing and so it’s not surprising that they won’t agree necessarily.

However, if you compare the p-value of the difference between means to a CI of the difference between means, they will always agree. You have to compare apples to apples!

April 14, 2018 at 8:54 pm

First of all, I love all your posts and you really do make people appreciate statistics by explaining it intuitively compared to theoretical approaches I’ve come across in university courses and other online resources. Please continue the fantastic work!!!

At the end, you mentioned how you prefer confidence intervals as they consider both “size and precision of the estimated effect”. I’m confused as to what exactly size and precision mean in this context. I’d appreciate an explanation with reference to specific numbers from the example above.

Second, do p-values lack both size and precision in determination of statistical significance?

Thanks, Devansh

April 17, 2018 at 11:41 am

Hi Devansh,

Thanks for the nice comments. I really appreciate them!

I really need to write a post specifically about this issue.

Let’s first assume that we conduct our study and find that the mean cost is 330.6 and that we are testing whether that is different than 260. Further suppose that we perform the the hypothesis test and obtain a p-value that is statistically significant. We can reject the null and conclude that population mean does not equal 260. And we can see our sample estimate is 330.6. So, that’s what we learn using p-values and the sample estimate.

Confidence intervals add to that information. We know that if we were to perform the experiment again, we’d get different results. How different? Is the true population mean likely to be close to 330.6 or further away? CIs help us answer these questions. The 95% CI is [267 394]. The true population value is likely to be within this range. That range spans 127 dollars.

However, let’s suppose we perform the experiment again but this time use a much larger sample size and obtain a mean of 351 and again a significant p-value. However, thanks to the large sample size, we obtain a 95 CI of [340 362]. Now we know that the population value is likely to fall within this much tighter interval of only 22 dollars. This estimate is much more precise.

Sometimes you can obtain a significant p-value for a result that is too imprecise to be useful. For example, with first CI, it might be too wide to be useful for what we need to do with our results. Maybe we’re helping people make budgets and that is too wide to allow for practical planning. However, the more precise estimate of the second study allows for better budgetary planning! That determination how much precision is required must be made using subject-area knowledge and focusing on the practical usage of the results. P-values don’t indicate the precision of the estimates in this manner!

I hope this helps clarify this precision issue!

Comments and Questions Cancel reply

Forgot your Password?

First, please create an account

Significance level and power of a hypothesis test.

Selecting an Appropriate Significance Level
Cautions about Significance Level
Power of a Hypothesis Test

1. Significance Level

Before we begin, you should first understand what is meant by statistical significance. When you calculate a test statistic in a hypothesis test, you can calculate the p-value. The p-value is the probability that you would have obtained a statistic as large (or small, or extreme) as the one you got if the null hypothesis is true. It's a conditional probability.

Sometimes you’re willing to attribute whatever difference you found between your statistic and your parameter to chance. If this is the case, you fail to reject the null hypothesis, if you’re willing to write off the differences between your statistic and your hypothesized parameter.

If you’re not, meaning it's just too far away from the mean to attribute to chance, then you’re going to reject the null hypothesis in favor of the alternative.

This is what it might look like for a two-tailed test.

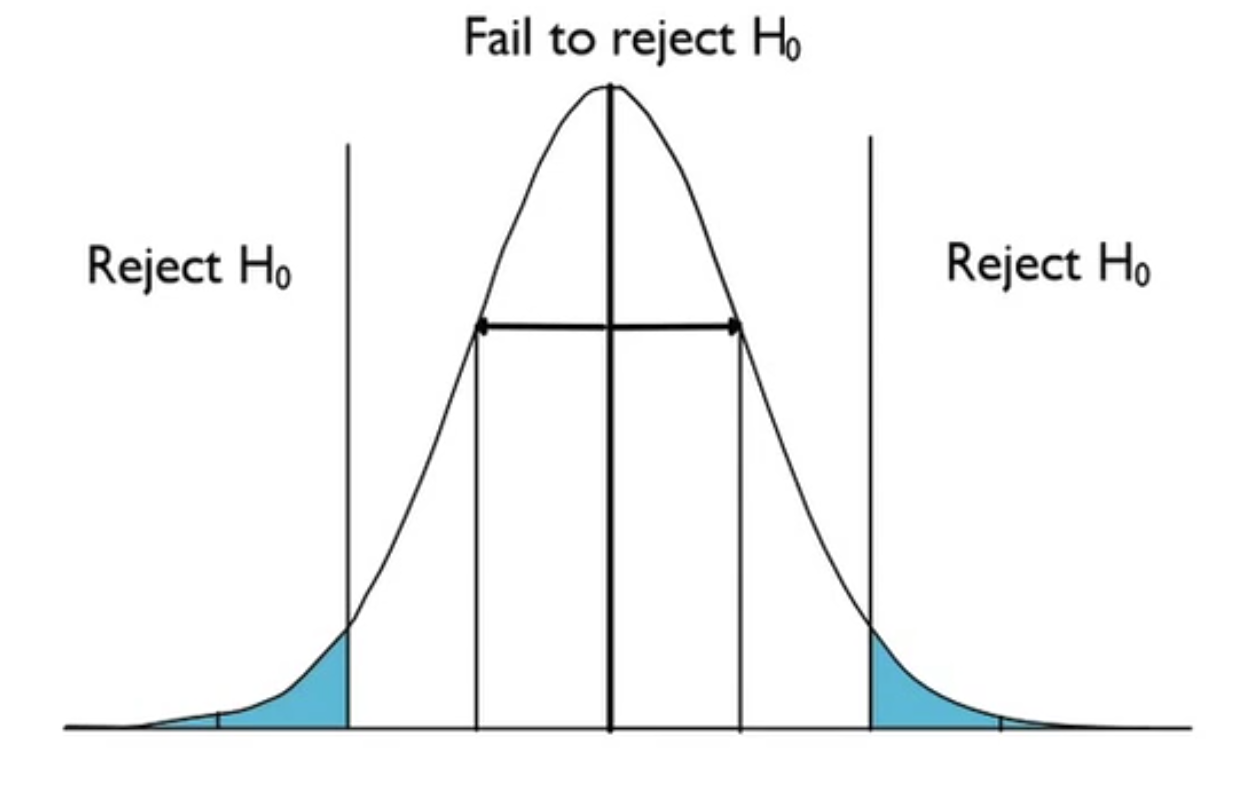
The hypothesized mean is right in the center of the normal distribution. Anything that is considered to be too far away--something like two standard deviations or more away--you would reject the null hypothesis. Anything you might attribute to chance, within the two standard deviations, you would fail to reject the null hypothesis. Again, this is assuming that the null hypothesis is true.

However, think about this. All of this curve assumes that the null hypothesis is true, but you make a decision to reject the null hypothesis anyway if the statistic you got is far away. It means that this would rarely happen by chance. But, it's still the wrong thing to do technically, if the null hypothesis is true. This idea that we're comfortable making some error sometimes is called a significance level.

The probability of rejecting the null hypothesis in error, in other words, rejecting the null hypothesis when it is, in fact, true, is called a Type I Error.

Fortunately, you get to choose how big you want this error to be. You could have stated that three standard deviations from the mean on either side as "too far away". Or, for instance, you could say you only want to be wrong 1% of the time, or 5% of the time, meaning that you are rejecting the null hypothesis in error that often.

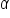
When you choose how big you want alpha to be, you do it before you start the tests. You do it this way to reduce bias because if you already ran the tests, you could choose an alpha level that would automatically make your result seem more significant than it is. You don't want to bias your results that way.

Take a look back at this visual here.

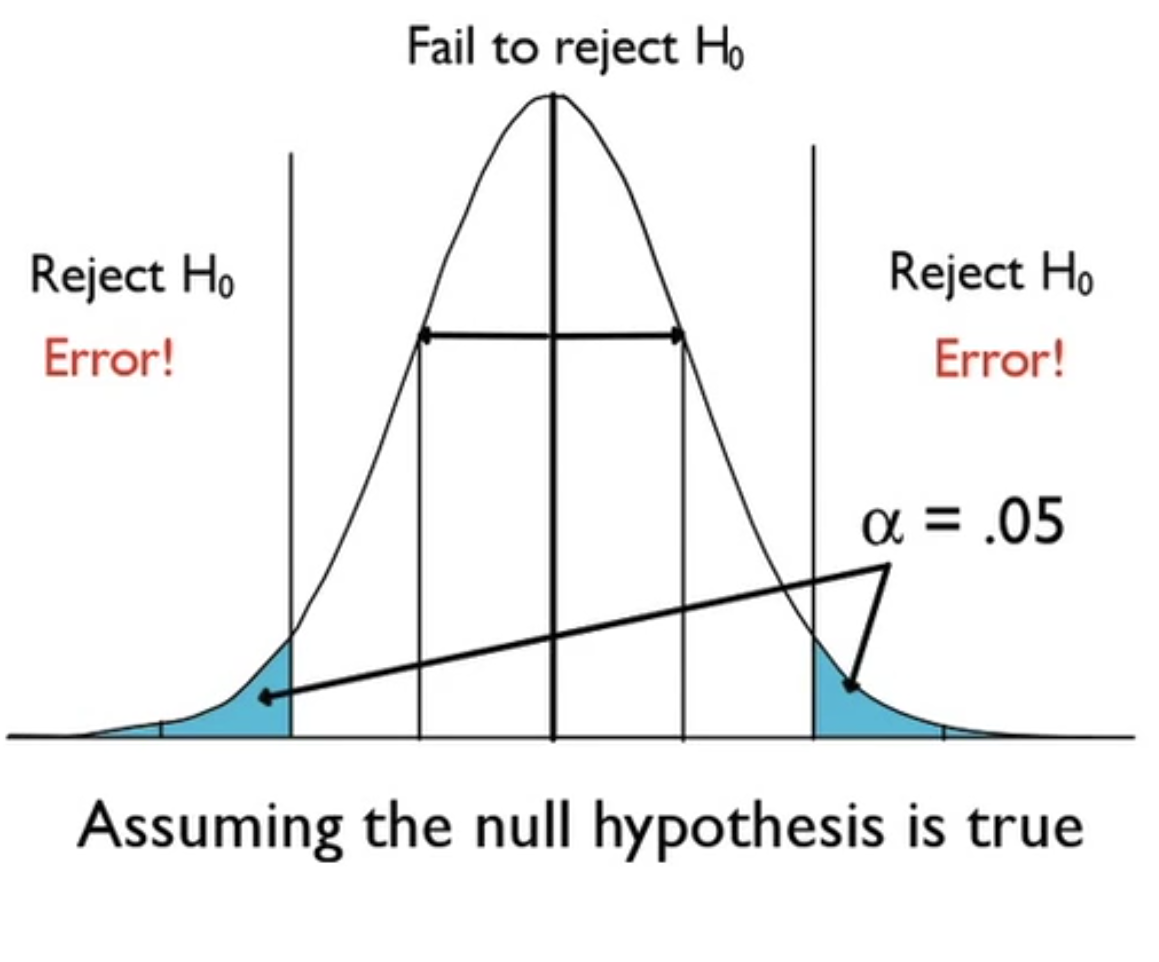
The alpha, in this case, is 0.05. If you recall, the 68-95-99.7 rule says that 95% of the values will fall within two standard deviations of the mean, meaning that 5% of the values will fall outside of those two standard deviations. Your decision to reject the null hypothesis will be 5% of the time; the most extreme 5% of cases, you will not be willing to attribute to chance variation from the hypothesized mean.

The level of significance will also depend on the type of experiment that you're doing.

If you want to be really cautious and not reject the null hypothesis in error very much, you'll choose a low significance level, like 0.01. This means that only the most extreme 1% of cases will have the null hypothesis rejected.

If you don't believe a Type I Error is going to be that bad, you might allow the significance level to be something higher, like 0.05 or 0.10. Those still seem like low numbers. However, think about what that means. This means that one out of every 20, or one out of every ten samples of that particular size will have the null hypothesis rejected even when it's true. Are you willing to make that mistake one out of every 20 times or once every ten times? Or are you only willing to make that mistake one out of every 100 times? Setting this value to something really low reduces the probability that you make that error.

It is important to note that you don't want the significance level to be too low. The problem with setting it really low is that as you lower the value of a Type 1 Error, you actually increase the probability of a Type II Error.

A Type II Error is failing to reject the null hypothesis when a difference does exist. This reduces the power or the sensitivity of your significance test, meaning that you will not be able to detect very real differences from the null hypothesis when they actually exist if your alpha level is set too low.

2. Power of a Hypothesis Test

You might wonder, what is power? Power is the ability of a hypothesis test to detect a difference that is present.

Consider the curves below. Note that μ 0 is the hypothesized mean and μ A is the actual mean. The actual mean is different than the null hypothesis; therefore, you should reject the null hypothesis. What you end up with is an identical curve to the original normal curve.

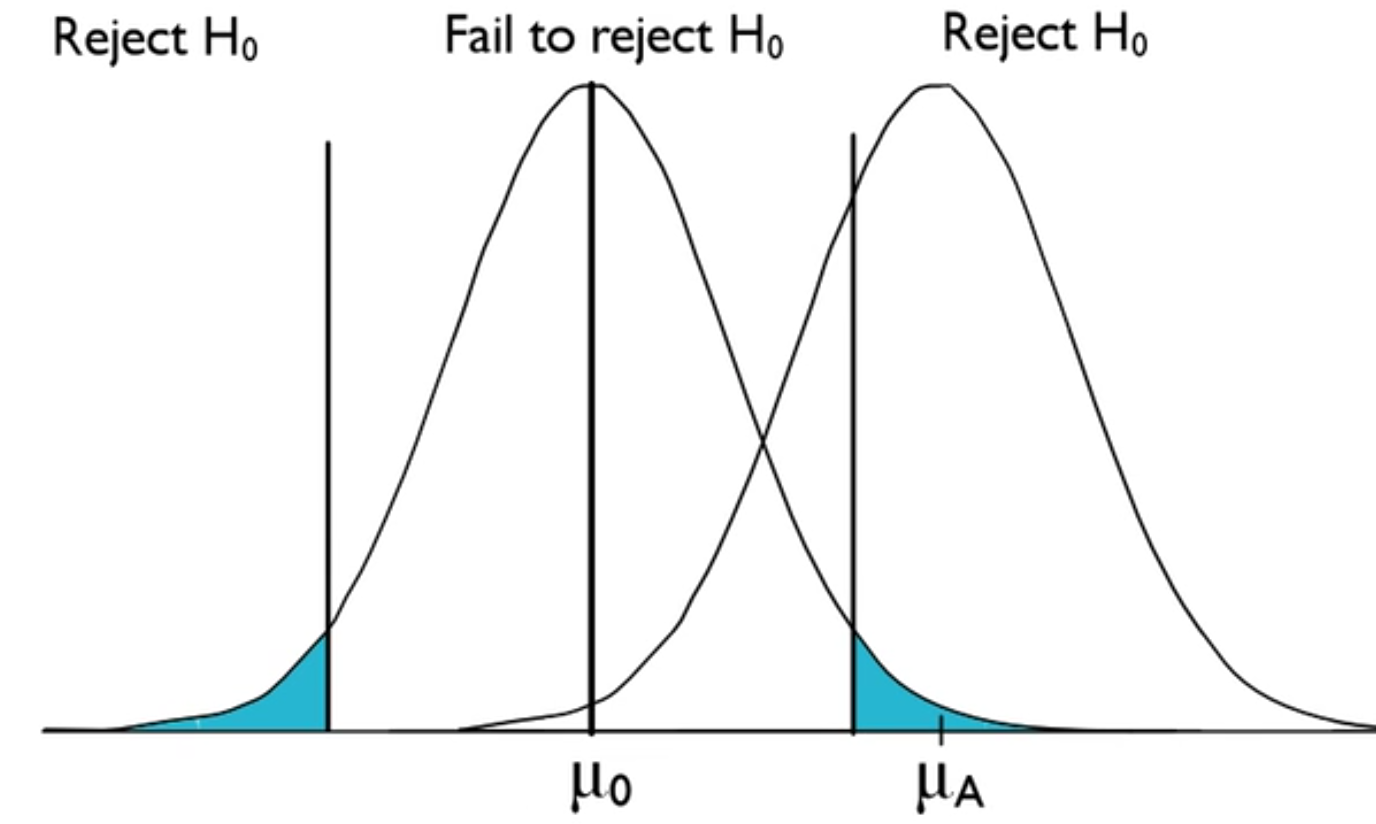
If you take a look at the curve below, it illustrates the way the data is actually behaving, versus the way you thought it should behave based on the null hypothesis. This line in the sand still exists, which means that because we should reject the null hypothesis, this area in orange is a mistake.

Failing to reject the null hypothesis is wrong, if this is actually the mean, which is different from the null hypothesis' mean. This is a type II error.

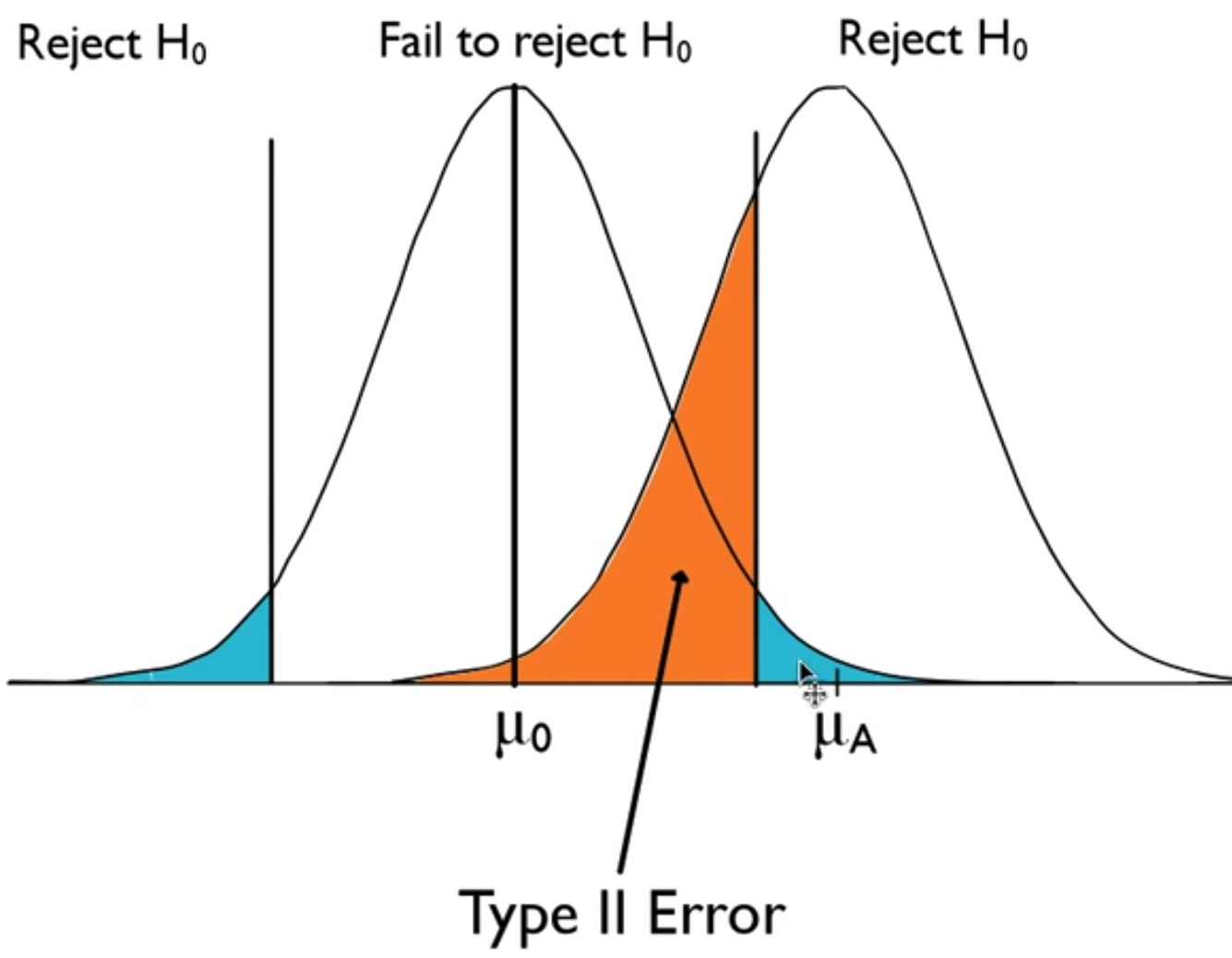
Now, the area in yellow on the other side, where you are correctly rejecting the null hypothesis when a difference is present, is called power of a hypothesis test . Power is the probability of rejecting the null hypothesis correctly, rejecting when the null hypothesis is false, which is a correct decision.

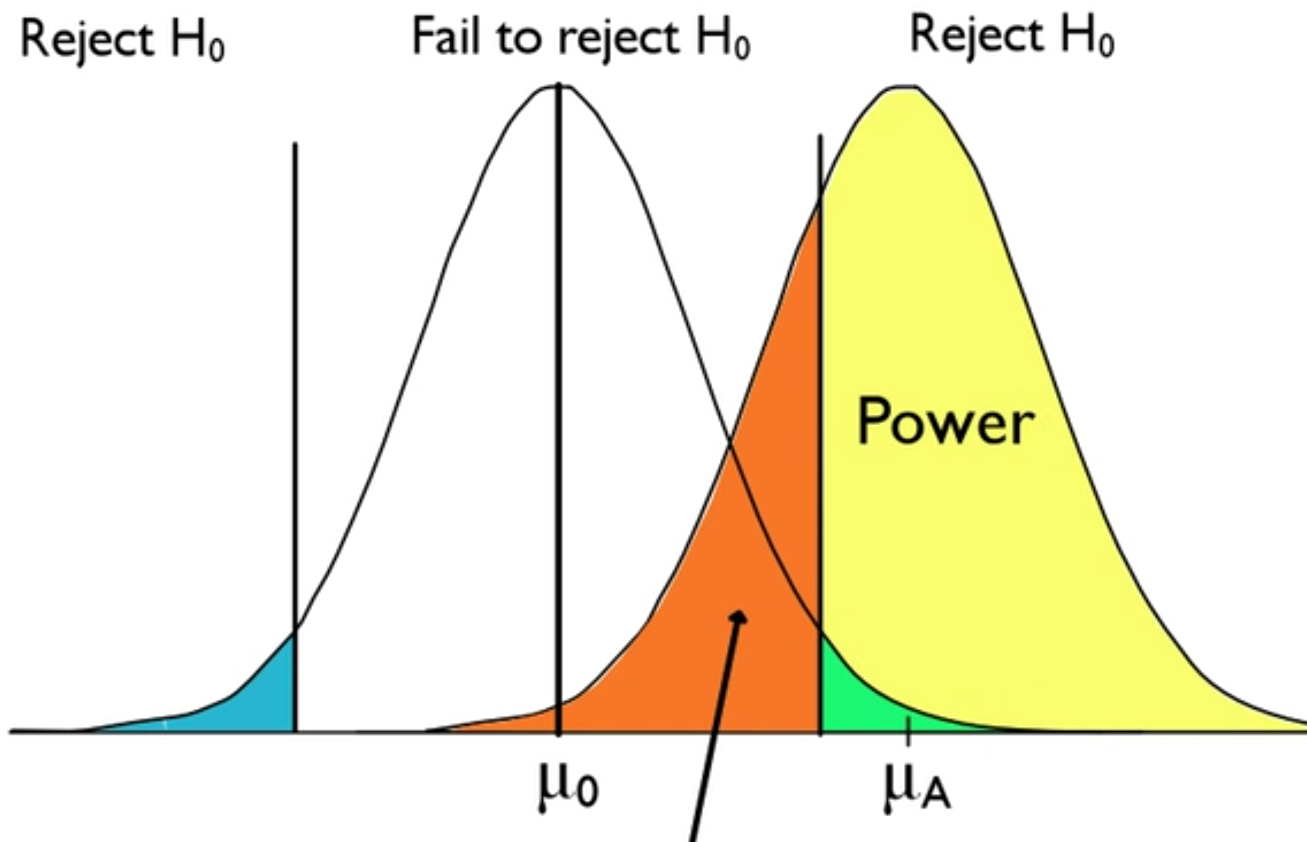
summary The probability of a type I error is a value that you get to choose in a hypothesis test. It is called the significance level and is denoted with the Greek letter alpha. Choosing a big significance level allows you to reject the null hypothesis more often, though the problem is that sometimes we reject the null hypothesis in error. When the difference really doesn't exist, you say that a difference does exist. However, if you choose a really small one, you reject the null hypothesis less often. Sometimes you fail to reject the null hypothesis in error as well. There's no foolproof method here. Usually, you want to keep your significance levels low, such as 0.05 or 0.01. Note that 0.05 is the default choice for most significance tests for most hypothesis testing. Good luck!

Source: Adapted from Sophia tutorial by Jonathan Osters.

The probability that we reject the null hypothesis (correctly) when a difference truly does exist.

open parentheses alpha close parentheses

Privacy Policy
Cookie Policy
Terms of Use

P-Value And Statistical Significance: What It Is & Why It Matters

Saul McLeod, PhD

Editor-in-Chief for Simply Psychology

BSc (Hons) Psychology, MRes, PhD, University of Manchester

Saul McLeod, PhD., is a qualified psychology teacher with over 18 years of experience in further and higher education. He has been published in peer-reviewed journals, including the Journal of Clinical Psychology.

Learn about our Editorial Process

Olivia Guy-Evans, MSc

Associate Editor for Simply Psychology

BSc (Hons) Psychology, MSc Psychology of Education

Olivia Guy-Evans is a writer and associate editor for Simply Psychology. She has previously worked in healthcare and educational sectors.

On This Page:

The p-value in statistics quantifies the evidence against a null hypothesis. A low p-value suggests data is inconsistent with the null, potentially favoring an alternative hypothesis. Common significance thresholds are 0.05 or 0.01.

P-Value Explained in Normal Distribution

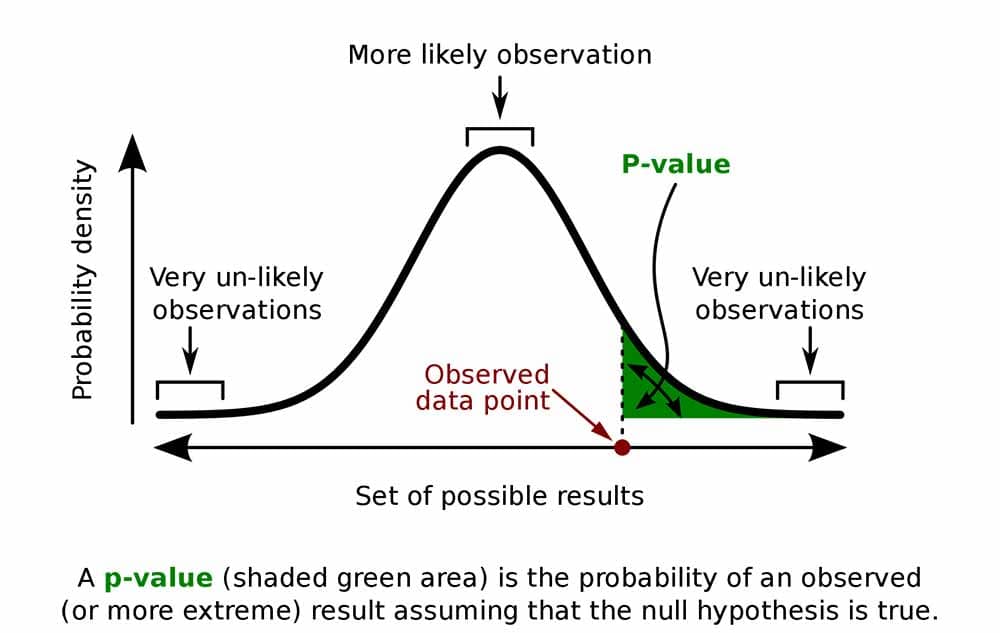
Hypothesis testing

When you perform a statistical test, a p-value helps you determine the significance of your results in relation to the null hypothesis.

The null hypothesis (H0) states no relationship exists between the two variables being studied (one variable does not affect the other). It states the results are due to chance and are not significant in supporting the idea being investigated. Thus, the null hypothesis assumes that whatever you try to prove did not happen.

The alternative hypothesis (Ha or H1) is the one you would believe if the null hypothesis is concluded to be untrue.

The alternative hypothesis states that the independent variable affected the dependent variable, and the results are significant in supporting the theory being investigated (i.e., the results are not due to random chance).

What a p-value tells you

A p-value, or probability value, is a number describing how likely it is that your data would have occurred by random chance (i.e., that the null hypothesis is true).

The level of statistical significance is often expressed as a p-value between 0 and 1.

The smaller the p -value, the less likely the results occurred by random chance, and the stronger the evidence that you should reject the null hypothesis.

Remember, a p-value doesn’t tell you if the null hypothesis is true or false. It just tells you how likely you’d see the data you observed (or more extreme data) if the null hypothesis was true. It’s a piece of evidence, not a definitive proof.

Example: Test Statistic and p-Value

Suppose you’re conducting a study to determine whether a new drug has an effect on pain relief compared to a placebo. If the new drug has no impact, your test statistic will be close to the one predicted by the null hypothesis (no difference between the drug and placebo groups), and the resulting p-value will be close to 1. It may not be precisely 1 because real-world variations may exist. Conversely, if the new drug indeed reduces pain significantly, your test statistic will diverge further from what’s expected under the null hypothesis, and the p-value will decrease. The p-value will never reach zero because there’s always a slim possibility, though highly improbable, that the observed results occurred by random chance.

P-value interpretation

The significance level (alpha) is a set probability threshold (often 0.05), while the p-value is the probability you calculate based on your study or analysis.

A p-value less than or equal to your significance level (typically ≤ 0.05) is statistically significant.

A p-value less than or equal to a predetermined significance level (often 0.05 or 0.01) indicates a statistically significant result, meaning the observed data provide strong evidence against the null hypothesis.

This suggests the effect under study likely represents a real relationship rather than just random chance.

For instance, if you set α = 0.05, you would reject the null hypothesis if your p -value ≤ 0.05.

It indicates strong evidence against the null hypothesis, as there is less than a 5% probability the null is correct (and the results are random).

Therefore, we reject the null hypothesis and accept the alternative hypothesis.

Example: Statistical Significance

Upon analyzing the pain relief effects of the new drug compared to the placebo, the computed p-value is less than 0.01, which falls well below the predetermined alpha value of 0.05. Consequently, you conclude that there is a statistically significant difference in pain relief between the new drug and the placebo.

What does a p-value of 0.001 mean?

A p-value of 0.001 is highly statistically significant beyond the commonly used 0.05 threshold. It indicates strong evidence of a real effect or difference, rather than just random variation.

Specifically, a p-value of 0.001 means there is only a 0.1% chance of obtaining a result at least as extreme as the one observed, assuming the null hypothesis is correct.

Such a small p-value provides strong evidence against the null hypothesis, leading to rejecting the null in favor of the alternative hypothesis.

A p-value more than the significance level (typically p > 0.05) is not statistically significant and indicates strong evidence for the null hypothesis.

This means we retain the null hypothesis and reject the alternative hypothesis. You should note that you cannot accept the null hypothesis; we can only reject it or fail to reject it.

Note : when the p-value is above your threshold of significance, it does not mean that there is a 95% probability that the alternative hypothesis is true.

One-Tailed Test

Probability and statistical significance in ab testing. Statistical significance in a b experiments

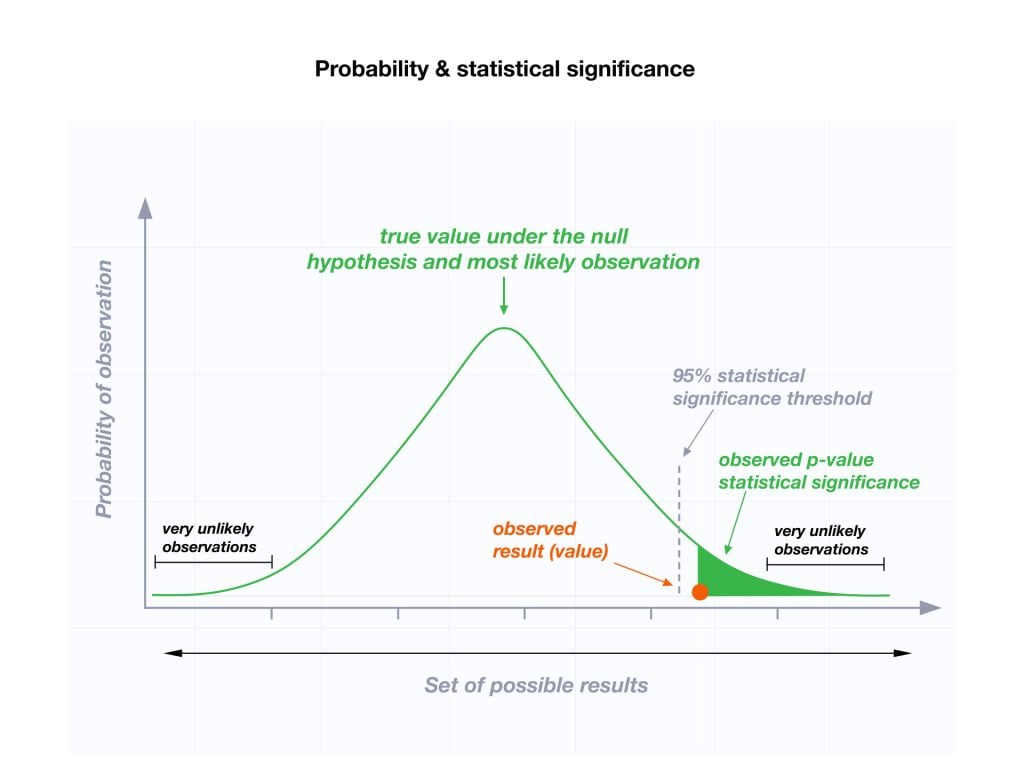
Two-Tailed Test

How do you calculate the p-value ?

Most statistical software packages like R, SPSS, and others automatically calculate your p-value. This is the easiest and most common way.

Online resources and tables are available to estimate the p-value based on your test statistic and degrees of freedom.

These tables help you understand how often you would expect to see your test statistic under the null hypothesis.

Understanding the Statistical Test:

Different statistical tests are designed to answer specific research questions or hypotheses. Each test has its own underlying assumptions and characteristics.

For example, you might use a t-test to compare means, a chi-squared test for categorical data, or a correlation test to measure the strength of a relationship between variables.

Be aware that the number of independent variables you include in your analysis can influence the magnitude of the test statistic needed to produce the same p-value.

This factor is particularly important to consider when comparing results across different analyses.

Example: Choosing a Statistical Test

If you’re comparing the effectiveness of just two different drugs in pain relief, a two-sample t-test is a suitable choice for comparing these two groups. However, when you’re examining the impact of three or more drugs, it’s more appropriate to employ an Analysis of Variance ( ANOVA) . Utilizing multiple pairwise comparisons in such cases can lead to artificially low p-values and an overestimation of the significance of differences between the drug groups.

How to report

A statistically significant result cannot prove that a research hypothesis is correct (which implies 100% certainty).

Instead, we may state our results “provide support for” or “give evidence for” our research hypothesis (as there is still a slight probability that the results occurred by chance and the null hypothesis was correct – e.g., less than 5%).

Example: Reporting the results

In our comparison of the pain relief effects of the new drug and the placebo, we observed that participants in the drug group experienced a significant reduction in pain ( M = 3.5; SD = 0.8) compared to those in the placebo group ( M = 5.2; SD = 0.7), resulting in an average difference of 1.7 points on the pain scale (t(98) = -9.36; p < 0.001).

The 6th edition of the APA style manual (American Psychological Association, 2010) states the following on the topic of reporting p-values:

“When reporting p values, report exact p values (e.g., p = .031) to two or three decimal places. However, report p values less than .001 as p < .001.

The tradition of reporting p values in the form p < .10, p < .05, p < .01, and so forth, was appropriate in a time when only limited tables of critical values were available.” (p. 114)

Do not use 0 before the decimal point for the statistical value p as it cannot equal 1. In other words, write p = .001 instead of p = 0.001.
Please pay attention to issues of italics ( p is always italicized) and spacing (either side of the = sign).
p = .000 (as outputted by some statistical packages such as SPSS) is impossible and should be written as p < .001.
The opposite of significant is “nonsignificant,” not “insignificant.”

Why is the p -value not enough?

A lower p-value is sometimes interpreted as meaning there is a stronger relationship between two variables.

However, statistical significance means that it is unlikely that the null hypothesis is true (less than 5%).

To understand the strength of the difference between the two groups (control vs. experimental) a researcher needs to calculate the effect size .

When do you reject the null hypothesis?

In statistical hypothesis testing, you reject the null hypothesis when the p-value is less than or equal to the significance level (α) you set before conducting your test. The significance level is the probability of rejecting the null hypothesis when it is true. Commonly used significance levels are 0.01, 0.05, and 0.10.

Remember, rejecting the null hypothesis doesn’t prove the alternative hypothesis; it just suggests that the alternative hypothesis may be plausible given the observed data.

The p -value is conditional upon the null hypothesis being true but is unrelated to the truth or falsity of the alternative hypothesis.

What does p-value of 0.05 mean?

If your p-value is less than or equal to 0.05 (the significance level), you would conclude that your result is statistically significant. This means the evidence is strong enough to reject the null hypothesis in favor of the alternative hypothesis.

Are all p-values below 0.05 considered statistically significant?

No, not all p-values below 0.05 are considered statistically significant. The threshold of 0.05 is commonly used, but it’s just a convention. Statistical significance depends on factors like the study design, sample size, and the magnitude of the observed effect.

A p-value below 0.05 means there is evidence against the null hypothesis, suggesting a real effect. However, it’s essential to consider the context and other factors when interpreting results.

Researchers also look at effect size and confidence intervals to determine the practical significance and reliability of findings.

How does sample size affect the interpretation of p-values?

Sample size can impact the interpretation of p-values. A larger sample size provides more reliable and precise estimates of the population, leading to narrower confidence intervals.

With a larger sample, even small differences between groups or effects can become statistically significant, yielding lower p-values. In contrast, smaller sample sizes may not have enough statistical power to detect smaller effects, resulting in higher p-values.

Therefore, a larger sample size increases the chances of finding statistically significant results when there is a genuine effect, making the findings more trustworthy and robust.

Can a non-significant p-value indicate that there is no effect or difference in the data?

No, a non-significant p-value does not necessarily indicate that there is no effect or difference in the data. It means that the observed data do not provide strong enough evidence to reject the null hypothesis.

There could still be a real effect or difference, but it might be smaller or more variable than the study was able to detect.

Other factors like sample size, study design, and measurement precision can influence the p-value. It’s important to consider the entire body of evidence and not rely solely on p-values when interpreting research findings.

Can P values be exactly zero?

While a p-value can be extremely small, it cannot technically be absolute zero. When a p-value is reported as p = 0.000, the actual p-value is too small for the software to display. This is often interpreted as strong evidence against the null hypothesis. For p values less than 0.001, report as p < .001

Further Information

P Value Calculator From T Score
P-Value Calculator For Chi-Square
P-values and significance tests (Kahn Academy)
Hypothesis testing and p-values (Kahn Academy)
Wasserstein, R. L., Schirm, A. L., & Lazar, N. A. (2019). Moving to a world beyond “ p “< 0.05”.
Criticism of using the “ p “< 0.05”.
Publication manual of the American Psychological Association
Statistics for Psychology Book Download

Bland, J. M., & Altman, D. G. (1994). One and two sided tests of significance: Authors’ reply. BMJ: British Medical Journal , 309 (6958), 874.

Goodman, S. N., & Royall, R. (1988). Evidence and scientific research. American Journal of Public Health , 78 (12), 1568-1574.

Goodman, S. (2008, July). A dirty dozen: twelve p-value misconceptions . In Seminars in hematology (Vol. 45, No. 3, pp. 135-140). WB Saunders.

Lang, J. M., Rothman, K. J., & Cann, C. I. (1998). That confounded P-value. Epidemiology (Cambridge, Mass.) , 9 (1), 7-8.

Hypothesis Testing with Z-Test: Significance Level and Rejection Region

Join over 2 million students who advanced their careers with 365 Data Science. Learn from instructors who have worked at Meta, Spotify, Google, IKEA, Netflix, and Coca-Cola and master Python, SQL, Excel, machine learning, data analysis, AI fundamentals, and more.

hypothesis test and level of significance

If you want to understand why hypothesis testing works, you should first have an idea about the significance level and the reject region . We assume you already know what a hypothesis is , so let’s jump right into the action.

What Is the Significance Level?

First, we must define the term significance level .

Normally, we aim to reject the null if it is false.

However, as with any test, there is a small chance that we could get it wrong and reject a null hypothesis that is true.

How Is the Significance Level Denoted?

The significance level is denoted by α and is the probability of rejecting the null hypothesis , if it is true.

α and is the probability of rejecting the null hypothesis, significance level

So, the probability of making this error.

Typical values for α are 0.01, 0.05 and 0.1. It is a value that we select based on the certainty we need. In most cases, the choice of α is determined by the context we are operating in, but 0.05 is the most commonly used value.

A Case in Point

Say, we need to test if a machine is working properly. We would expect the test to make little or no mistakes. As we want to be very precise, we should pick a low significance level such as 0.01.

The famous Coca Cola glass bottle is 12 ounces. If the machine pours 12.1 ounces, some of the liquid would be spilled, and the label would be damaged as well. So, in certain situations, we need to be as accurate as possible.

Higher Degree of Error

However, if we are analyzing humans or companies, we would expect more random or at least uncertain behavior. Hence, a higher degree of error.

You expect more random behavior, significance level

For instance, if we want to predict how much Coca Cola its consumers drink on average, the difference between 12 ounces and 12.1 ounces will not be that crucial. So, we can choose a higher significance level like 0.05 or 0.1.

The difference between 12 and 12.1, significance level

Hypothesis Testing: Performing a Z-Test

Now that we have an idea about the significance level , let’s get to the mechanics of hypothesis testing.

Imagine you are consulting a university and want to carry out an analysis on how students are performing on average.

How students are performing on average, significance-level

The university dean believes that on average students have a GPA of 70%. Being the data-driven researcher that you are, you can’t simply agree with his opinion, so you start testing.

The null hypothesis is: The population mean grade is 70%.

This is a hypothesized value.

The alternative hypothesis is: The population mean grade is not 70%. You can see how both of them are denoted, below.

University Dean example: Null hypothesis equals the population mean

Visualizing the Grades

Assuming that the population of grades is normally distributed, all grades received by students should look in the following way.

Distribution of grades, significance level

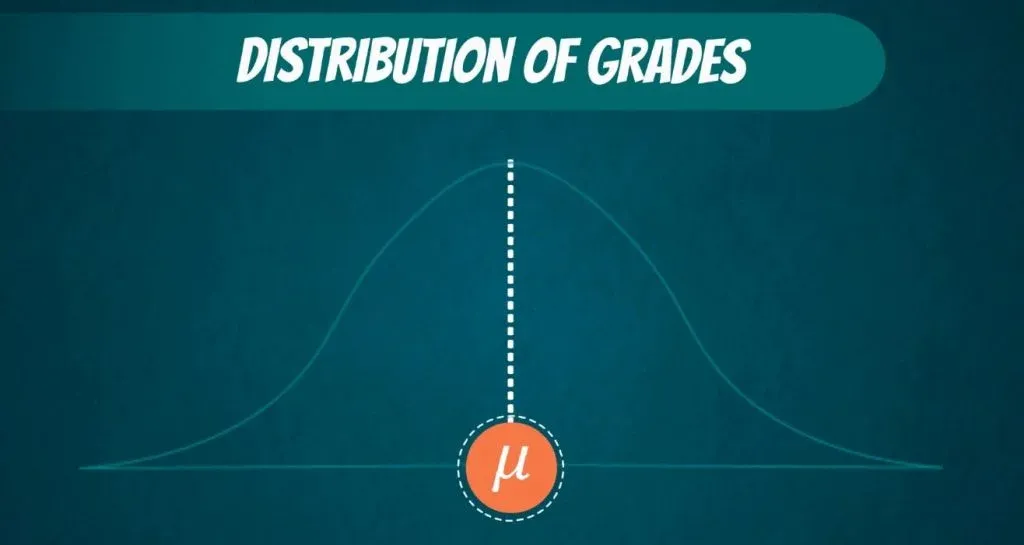
That is the true population mean .

Performing a Z-test

Now, a test we would normally perform is the Z-test . The formula is:

Z equals the sample mean , minus the hypothesized mean , divided by the standard error .

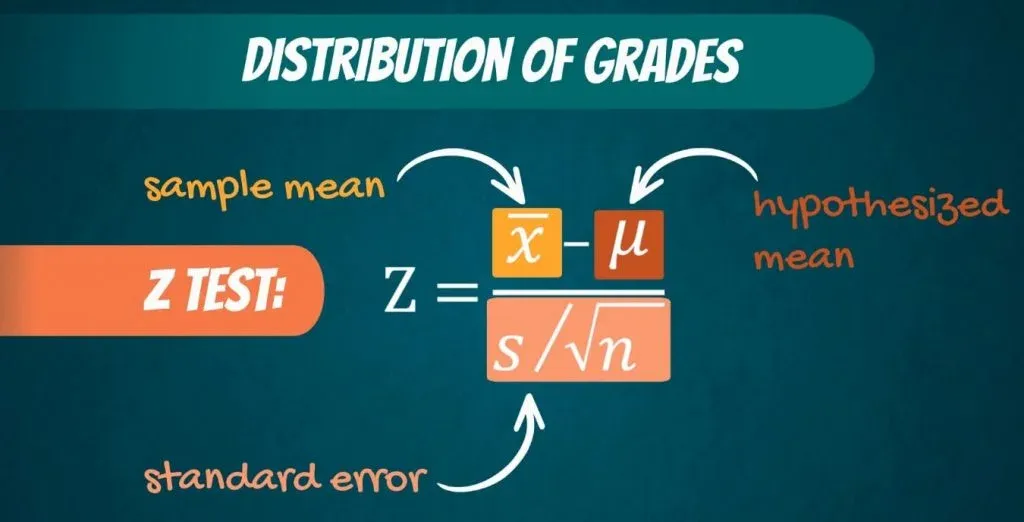
The idea is the following.

We are standardizing or scaling the sample mean we got. (You can quickly obtain it with our Mean, Median, Mode calculator .) If the sample mean is close enough to the hypothesized mean , then Z will be close to 0. Otherwise, it will be far away from it. Naturally, if the sample mean is exactly equal to the hypothesized mean , Z will be 0.

If the sample mean is exactly equal to the hypothesized mean, Z will be 0, significance level

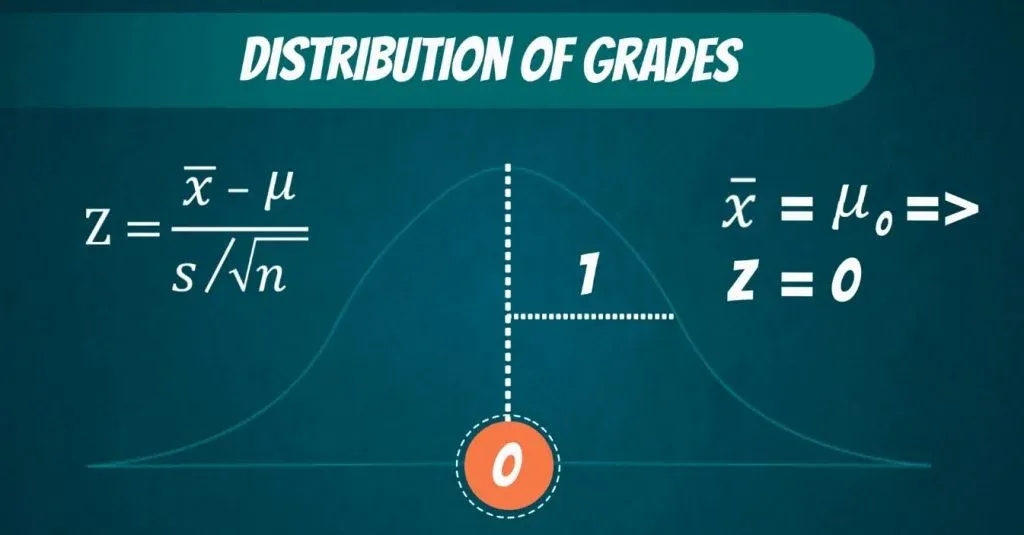
In all these cases, we would accept the null hypothesis .

What Is the Rejection Region?

The question here is the following:

How big should Z be for us to reject the null hypothesis ?

Well, there is a cut-off line. Since we are conducting a two-sided or a two-tailed test, there are two cut-off lines, one on each side.

Distribution of Z (standard normal distribution), significance level

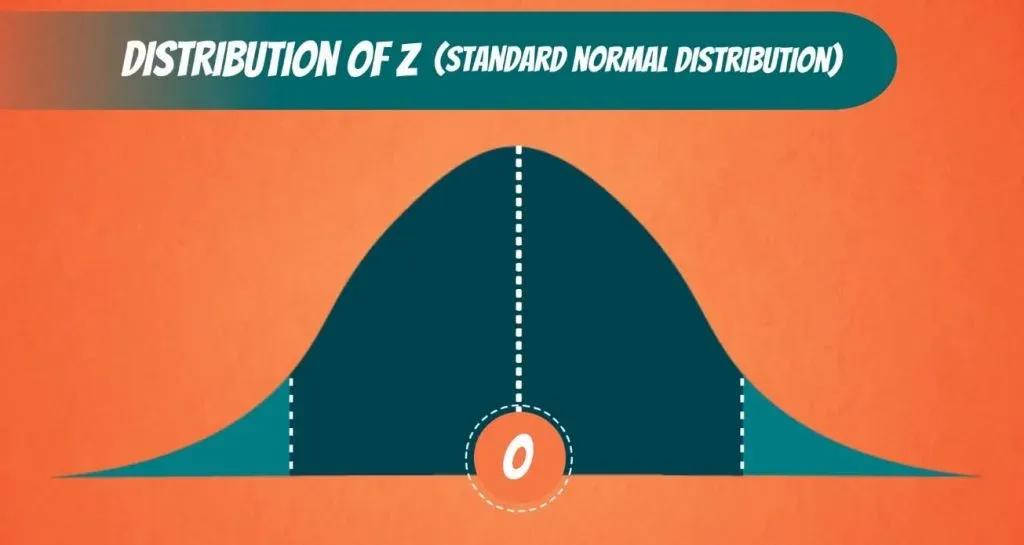
When we calculate Z , we will get a value. If this value falls into the middle part, then we cannot reject the null. If it falls outside, in the shaded region, then we reject the null hypothesis .

That is why the shaded part is called: rejection region , as you can see below.

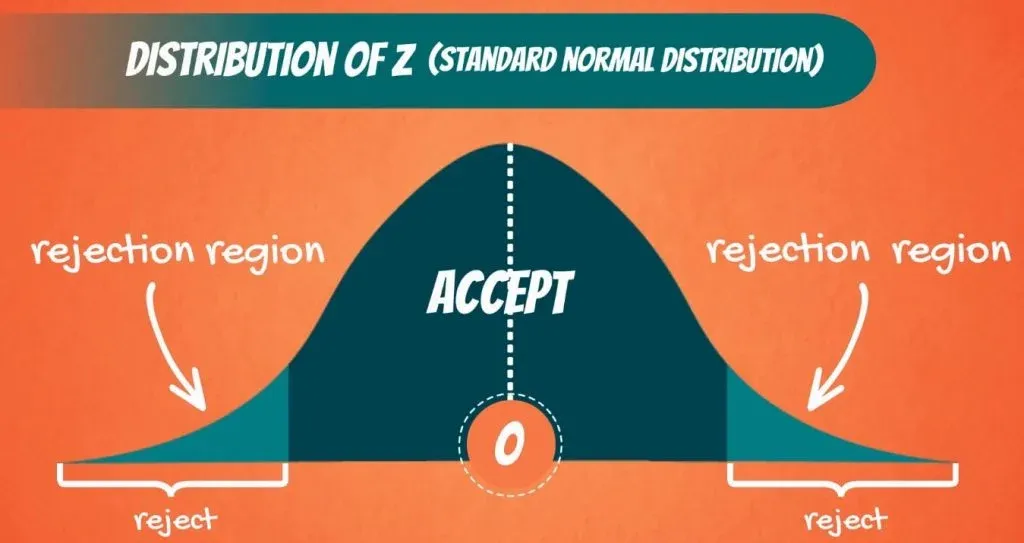
What Does the Rejection Region Depend on?

The area that is cut-off actually depends on the significance level .

Say the level of significance , α , is 0.05. Then we have α divided by 2, or 0.025 on the left side and 0.025 on the right side.

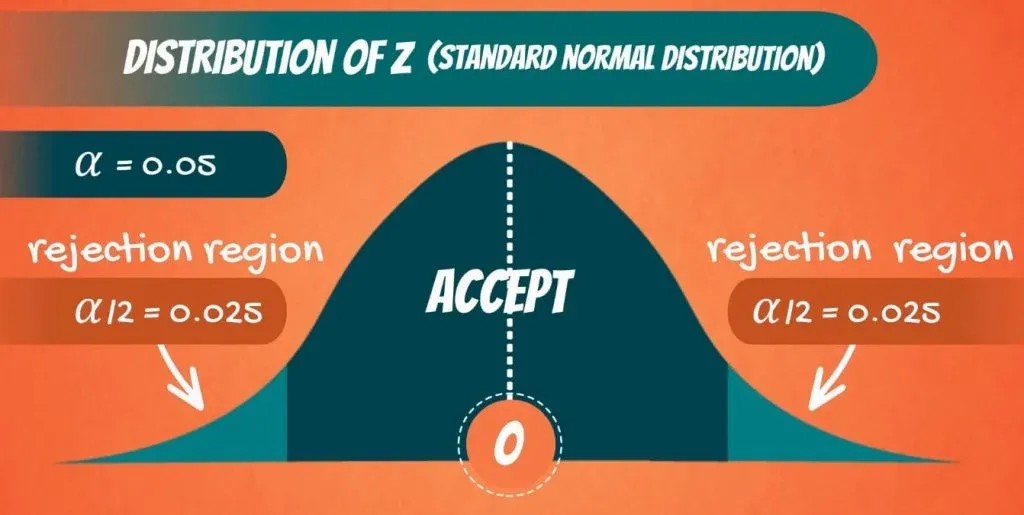
Now these are values we can check from the z-table . When α is 0.025, Z is 1.96. So, 1.96 on the right side and minus 1.96 on the left side.

Therefore, if the value we get for Z from the test is lower than minus 1.96, or higher than 1.96, we will reject the null hypothesis . Otherwise, we will accept it.

That’s more or less how hypothesis testing works.

We scale the sample mean with respect to the hypothesized value. If Z is close to 0, then we cannot reject the null. If it is far away from 0, then we reject the null hypothesis .

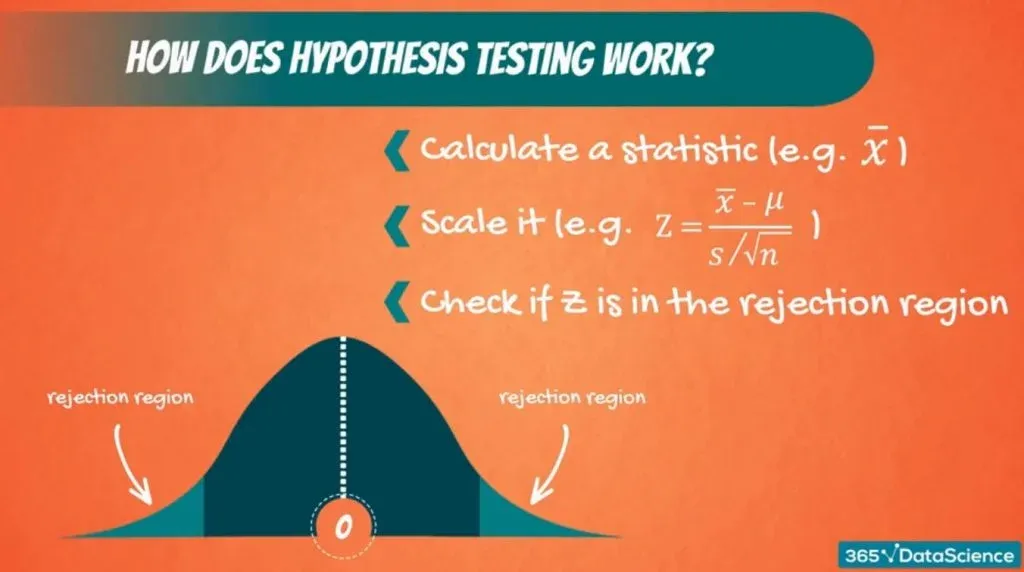
Example of One Tailed Test

What about one-sided tests? We have those too!

Let’s consider the following situation.

Paul says data scientists earn more than $125,000. So, H 0 is: μ 0 is bigger than $125,000.

The alternative is that μ 0 is lower or equal to 125,000.

Using the same significance level , this time, the whole rejection region is on the left. So, the rejection region has an area of α . Looking at the z-table, that corresponds to a Z -score of 1.645. Since it is on the left, it is with a minus sign.

Accept or Reject

Now, when calculating our test statistic Z , if we get a value lower than -1.645, we would reject the null hypothesis . We do that because we have statistical evidence that the data scientist salary is less than $125,000. Otherwise, we would accept it.

One-sided test: Z score is - 1.645 - rejecting null hypothesis

Another One-Tailed Test

To exhaust all possibilities, let’s explore another one-tailed test.

Say the university dean told you that the average GPA students get is lower than 70%. In that case, the null hypothesis is:

μ 0 is lower than 70%.

While the alternative is:

μ 0` is bigger or equal to 70%.

University Dean example: Null hypothesis lower than the population mean

In this situation, the rejection region is on the right side. So, if the test statistic is bigger than the cut-off z-score, we would reject the null, otherwise, we wouldn’t.

One-sided test: test statistic is bigger than the cut-off z-score - reject the null hypothesis

Importance of the Significance Level and the Rejection Region

To sum up, the significance level and the reject region are quite crucial in the process of hypothesis testing. The level of significance conducts the accuracy of prediction. We (the researchers) choose it depending on how big of a difference a possible error could make. On the other hand, the reject region helps us decide whether or not to reject the null hypothesis . After reading this and putting both of them into use, you will realize how convenient they make your work.

Interested in taking your skills from good to great? Try statistics course for free !

Next Tutorial: Providing a Few Linear Regression Examples

World-Class

Data Science

Learn with instructors from:

Iliya Valchanov

Co-founder of 365 Data Science

Iliya is a finance graduate with a strong quantitative background who chose the exciting path of a startup entrepreneur. He demonstrated a formidable affinity for numbers during his childhood, winning more than 90 national and international awards and competitions through the years. Iliya started teaching at university, helping other students learn statistics and econometrics. Inspired by his first happy students, he co-founded 365 Data Science to continue spreading knowledge. He authored several of the program’s online courses in mathematics, statistics, machine learning, and deep learning.

We Think you'll also like

Statistics Tutorials

Hypothesis Testing: Null Hypothesis and Alternative Hypothesis

False Positive vs. False Negative: Type I and Type II Errors in Statistical Hypothesis Testing

Calculating and Using Covariance and Linear Correlation Coefficient

Examples of Numerical and Categorical Variables

Hypothesis Testing for Means & Proportions

1
| 2
| 3
| 4
| 5
| 6
| 7
| 8
| 9
| 10

Hypothesis Testing: Upper-, Lower, and Two Tailed Tests

Type i and type ii errors.

All Modules

Z score Table

t score Table

The procedure for hypothesis testing is based on the ideas described above. Specifically, we set up competing hypotheses, select a random sample from the population of interest and compute summary statistics. We then determine whether the sample data supports the null or alternative hypotheses. The procedure can be broken down into the following five steps.

Step 1. Set up hypotheses and select the level of significance α.

H 0 : Null hypothesis (no change, no difference);

H 1 : Research hypothesis (investigator's belief); α =0.05

Upper-tailed, Lower-tailed, Two-tailed Tests

The research or alternative hypothesis can take one of three forms. An investigator might believe that the parameter has increased, decreased or changed. For example, an investigator might hypothesize:

: μ > μ , where μ is the comparator or null value (e.g., μ =191 in our example about weight in men in 2006) and an increase is hypothesized - this type of test is called an ; : μ < μ , where a decrease is hypothesized and this is called a ; or : μ ≠ μ where a difference is hypothesized and this is called a .

The exact form of the research hypothesis depends on the investigator's belief about the parameter of interest and whether it has possibly increased, decreased or is different from the null value. The research hypothesis is set up by the investigator before any data are collected.

Step 2. Select the appropriate test statistic.

The test statistic is a single number that summarizes the sample information. An example of a test statistic is the Z statistic computed as follows:

When the sample size is small, we will use t statistics (just as we did when constructing confidence intervals for small samples). As we present each scenario, alternative test statistics are provided along with conditions for their appropriate use.

Step 3. Set up decision rule.

The decision rule is a statement that tells under what circumstances to reject the null hypothesis. The decision rule is based on specific values of the test statistic (e.g., reject H 0 if Z > 1.645). The decision rule for a specific test depends on 3 factors: the research or alternative hypothesis, the test statistic and the level of significance. Each is discussed below.

The decision rule depends on whether an upper-tailed, lower-tailed, or two-tailed test is proposed. In an upper-tailed test the decision rule has investigators reject H 0 if the test statistic is larger than the critical value. In a lower-tailed test the decision rule has investigators reject H 0 if the test statistic is smaller than the critical value. In a two-tailed test the decision rule has investigators reject H 0 if the test statistic is extreme, either larger than an upper critical value or smaller than a lower critical value.
The exact form of the test statistic is also important in determining the decision rule. If the test statistic follows the standard normal distribution (Z), then the decision rule will be based on the standard normal distribution. If the test statistic follows the t distribution, then the decision rule will be based on the t distribution. The appropriate critical value will be selected from the t distribution again depending on the specific alternative hypothesis and the level of significance.
The third factor is the level of significance. The level of significance which is selected in Step 1 (e.g., α =0.05) dictates the critical value. For example, in an upper tailed Z test, if α =0.05 then the critical value is Z=1.645.

The following figures illustrate the rejection regions defined by the decision rule for upper-, lower- and two-tailed Z tests with α=0.05. Notice that the rejection regions are in the upper, lower and both tails of the curves, respectively. The decision rules are written below each figure.

Rejection Region for Upper-Tailed Z Test (H : μ > μ ) with α=0.05

The decision rule is: Reject H if Z 1.645.


α	Z
0.10	1.282
0.05	1.645
0.025	1.960
0.010	2.326
0.005	2.576
0.001	3.090
0.0001	3.719

Standard normal distribution with lower tail at -1.645 and alpha=0.05

Rejection Region for Lower-Tailed Z Test (H 1 : μ < μ 0 ) with α =0.05

The decision rule is: Reject H 0 if Z < 1.645.


a	Z
0.10	-1.282
0.05	-1.645
0.025	-1.960
0.010	-2.326
0.005	-2.576
0.001	-3.090
0.0001	-3.719

Standard normal distribution with two tails

Rejection Region for Two-Tailed Z Test (H 1 : μ ≠ μ 0 ) with α =0.05

The decision rule is: Reject H 0 if Z < -1.960 or if Z > 1.960.



0.20	1.282
0.10	1.645
0.05	1.960
0.010	2.576
0.001	3.291
0.0001	3.819

The complete table of critical values of Z for upper, lower and two-tailed tests can be found in the table of Z values to the right in "Other Resources."

Critical values of t for upper, lower and two-tailed tests can be found in the table of t values in "Other Resources."

Step 4. Compute the test statistic.

Here we compute the test statistic by substituting the observed sample data into the test statistic identified in Step 2.

Step 5. Conclusion.

The final conclusion is made by comparing the test statistic (which is a summary of the information observed in the sample) to the decision rule. The final conclusion will be either to reject the null hypothesis (because the sample data are very unlikely if the null hypothesis is true) or not to reject the null hypothesis (because the sample data are not very unlikely).

If the null hypothesis is rejected, then an exact significance level is computed to describe the likelihood of observing the sample data assuming that the null hypothesis is true. The exact level of significance is called the p-value and it will be less than the chosen level of significance if we reject H 0 .

Statistical computing packages provide exact p-values as part of their standard output for hypothesis tests. In fact, when using a statistical computing package, the steps outlined about can be abbreviated. The hypotheses (step 1) should always be set up in advance of any analysis and the significance criterion should also be determined (e.g., α =0.05). Statistical computing packages will produce the test statistic (usually reporting the test statistic as t) and a p-value. The investigator can then determine statistical significance using the following: If p < α then reject H 0 .

Step 1. Set up hypotheses and determine level of significance

H 0 : μ = 191 H 1 : μ > 191 α =0.05

The research hypothesis is that weights have increased, and therefore an upper tailed test is used.

Step 2. Select the appropriate test statistic.

Because the sample size is large (n > 30) the appropriate test statistic is

Step 3. Set up decision rule.

In this example, we are performing an upper tailed test (H 1 : μ> 191), with a Z test statistic and selected α =0.05. Reject H 0 if Z > 1.645.

We now substitute the sample data into the formula for the test statistic identified in Step 2.

We reject H 0 because 2.38 > 1.645. We have statistically significant evidence at a =0.05, to show that the mean weight in men in 2006 is more than 191 pounds. Because we rejected the null hypothesis, we now approximate the p-value which is the likelihood of observing the sample data if the null hypothesis is true. An alternative definition of the p-value is the smallest level of significance where we can still reject H 0 . In this example, we observed Z=2.38 and for α=0.05, the critical value was 1.645. Because 2.38 exceeded 1.645 we rejected H 0 . In our conclusion we reported a statistically significant increase in mean weight at a 5% level of significance. Using the table of critical values for upper tailed tests, we can approximate the p-value. If we select α=0.025, the critical value is 1.96, and we still reject H 0 because 2.38 > 1.960. If we select α=0.010 the critical value is 2.326, and we still reject H 0 because 2.38 > 2.326. However, if we select α=0.005, the critical value is 2.576, and we cannot reject H 0 because 2.38 < 2.576. Therefore, the smallest α where we still reject H 0 is 0.010. This is the p-value. A statistical computing package would produce a more precise p-value which would be in between 0.005 and 0.010. Here we are approximating the p-value and would report p < 0.010.

In all tests of hypothesis, there are two types of errors that can be committed. The first is called a Type I error and refers to the situation where we incorrectly reject H 0 when in fact it is true. This is also called a false positive result (as we incorrectly conclude that the research hypothesis is true when in fact it is not). When we run a test of hypothesis and decide to reject H 0 (e.g., because the test statistic exceeds the critical value in an upper tailed test) then either we make a correct decision because the research hypothesis is true or we commit a Type I error. The different conclusions are summarized in the table below. Note that we will never know whether the null hypothesis is really true or false (i.e., we will never know which row of the following table reflects reality).

Table - Conclusions in Test of Hypothesis


is True	Correct Decision	Type I Error
is False	Type II Error	Correct Decision

In the first step of the hypothesis test, we select a level of significance, α, and α= P(Type I error). Because we purposely select a small value for α, we control the probability of committing a Type I error. For example, if we select α=0.05, and our test tells us to reject H 0 , then there is a 5% probability that we commit a Type I error. Most investigators are very comfortable with this and are confident when rejecting H 0 that the research hypothesis is true (as it is the more likely scenario when we reject H 0 ).

When we run a test of hypothesis and decide not to reject H 0 (e.g., because the test statistic is below the critical value in an upper tailed test) then either we make a correct decision because the null hypothesis is true or we commit a Type II error. Beta (β) represents the probability of a Type II error and is defined as follows: β=P(Type II error) = P(Do not Reject H 0 | H 0 is false). Unfortunately, we cannot choose β to be small (e.g., 0.05) to control the probability of committing a Type II error because β depends on several factors including the sample size, α, and the research hypothesis. When we do not reject H 0 , it may be very likely that we are committing a Type II error (i.e., failing to reject H 0 when in fact it is false). Therefore, when tests are run and the null hypothesis is not rejected we often make a weak concluding statement allowing for the possibility that we might be committing a Type II error. If we do not reject H 0 , we conclude that we do not have significant evidence to show that H 1 is true. We do not conclude that H 0 is true.

The most common reason for a Type II error is a small sample size.

return to top | previous page | next page

Math Article
Level Of Significance

Level of Significance

Statistics is a branch of Mathematics. It deals with gathering, presenting, analyzing, organizing and interpreting the data, which is usually numerical. It is applied to many industrial, scientific, social and economic areas. While a researcher performs research, a hypothesis has to be set, which is known as the null hypothesis . This hypothesis is required to be tested via pre-defined statistical examinations. This process is termed as statistical hypothesis testing. The level of significance or Statistical significance is an important terminology that is quite commonly used in Statistics. In this article, we are going to discuss the level of significance in detail.

What is Statistical Significance?

In Statistics, “significance” means “not by chance” or “probably true”. We can say that if a statistician declares that some result is “highly significant”, then he indicates by stating that it might be very probably true. It does not mean that the result is highly significant, but it suggests that it is highly probable.

Level of Significance Definition

The level of significance is defined as the fixed probability of wrong elimination of null hypothesis when in fact, it is true. The level of significance is stated to be the probability of type I error and is preset by the researcher with the outcomes of error. The level of significance is the measurement of the statistical significance. It defines whether the null hypothesis is assumed to be accepted or rejected. It is expected to identify if the result is statistically significant for the null hypothesis to be false or rejected.

Level of Significance Symbol

The level of significance is denoted by the Greek symbol α (alpha). Therefore, the level of significance is defined as follows:

Significance Level = p (type I error) = α

The values or the observations are less likely when they are farther than the mean. The results are written as “significant at x%”.

Example: The value significant at 5% refers to p-value is less than 0.05 or p < 0.05. Similarly, significant at the 1% means that the p-value is less than 0.01.

The level of significance is taken at 0.05 or 5%. When the p-value is low, it means that the recognised values are significantly different from the population value that was hypothesised in the beginning. The p-value is said to be more significant if it is as low as possible. Also, the result would be highly significant if the p-value is very less. But, most generally, p-values smaller than 0.05 are known as significant, since getting a p-value less than 0.05 is quite a less practice.

How to Find the Level of Significance?

To measure the level of statistical significance of the result, the investigator first needs to calculate the p-value. It defines the probability of identifying an effect which provides that the null hypothesis is true. When the p-value is less than the level of significance (α), the null hypothesis is rejected. If the p-value so observed is not less than the significance level α, then theoretically null hypothesis is accepted. But practically, we often increase the size of the sample size and check if we reach the significance level. The general interpretation of the p-value based upon the level of significance of 10%:

If p > 0.1, then there will be no assumption for the null hypothesis
If p > 0.05 and p ≤ 0.1, it means that there will be a low assumption for the null hypothesis.
If p > 0.01 and p ≤ 0.05, then there must be a strong assumption about the null hypothesis.
If p ≤ 0.01, then a very strong assumption about the null hypothesis is indicated.

Level of Significance Example

If we obtain a p-value equal to 0.03, then it indicates that there are just 3% chances of getting a difference larger than that in our research, given that the null hypothesis exists. Now, we need to determine if this result is statistically significant enough.

We know that if the chances are 5% or less than that, then the null hypothesis is true, and we will tend to reject the null hypothesis and accept the alternative hypothesis. Here, in this case, the chances are 0.03, i.e. 3% (less than 5%), which eventually means that we will eliminate our null hypothesis and will accept an alternative hypothesis.

MATHS Related Links

Register with BYJU'S & Download Free PDFs

All Subjects

6.2 Type I and Type II errors, significance level, and power

3 min read • august 16, 2024

In hypothesis testing, we grapple with two key errors: Type I (false positives) and Type II (false negatives). These errors, along with significance levels and power , form the backbone of statistical decision-making. Understanding their interplay is crucial for interpreting research results and making informed choices.

Balancing these errors is a delicate act. Significance levels set the bar for Type I errors, while power measures our ability to detect true effects. Researchers must weigh the consequences of each error type, considering factors like sample size and effect size to design robust studies and draw meaningful conclusions.

Type I vs Type II Errors

Definitions and characteristics.

Type I error rejects a true null hypothesis resulting in a false positive
Type II error fails to reject a false null hypothesis resulting in a false negative
Probability of Type I error denoted by α (alpha)
Probability of Type II error denoted by β (beta)
Mutually exclusive and exhaustive outcomes in hypothesis testing when null hypothesis is false
Consequences vary depending on study context (medical research, product testing)
Critical for interpreting research results and making informed decisions

Examples and Implications

Medical testing: Type I error diagnoses healthy patient with disease, Type II error fails to diagnose sick patient (cancer screening)
Criminal justice: Type I error convicts innocent person, Type II error acquits guilty person
Quality control: Type I error rejects good product batch, Type II error accepts defective batch
Implications depend on field (pharmaceutical trials, environmental regulations)
Balancing errors crucial for effective decision-making in research and policy

Significance Level and Type I Errors

Relationship and interpretation.

Significance level (α) represents maximum acceptable probability of Type I error
Typically set before conducting hypothesis test (common values 0.05, 0.01)
Lower significance level reduces Type I error probability but increases Type II error risk
P-value compared to significance level for hypothesis test decisions
Direct relationship between significance level and Type I error probability
Critical for determining appropriate significance level in different research contexts

Application in Research

Medical research often uses lower significance levels (0.01) due to serious consequences of false positives
Social sciences may use higher significance levels (0.05 or 0.10) to balance Type I and Type II errors
Multiple comparisons problem requires adjusting significance level (Bonferroni correction)
Significance level affects confidence interval width (lower α leads to wider intervals)
Researchers must justify chosen significance level based on study goals and potential impacts

Power of Hypothesis Tests

Factors influencing power.

Power defined as probability of correctly rejecting false null hypothesis (1 - β)
Sample size positively correlates with power (larger samples provide more information)
Effect size positively influences power (larger differences easier to detect)
Significance level (α) affects power (higher α increases power but raises Type I error risk)
Data variability inversely related to power (lower standard deviation increases power)
Test directionality impacts power (one-tailed tests more powerful than two-tailed tests)

Power Analysis and Applications

A priori power analysis determines required sample size for desired power level
Post hoc power analysis assesses achieved power after study completion
Power analysis software tools available (G*Power, R packages)
Rule of thumb: 80% power often considered acceptable minimum
Power calculations crucial for grant proposals and study design justification
Low-powered studies risk missing true effects (Type II errors) and overestimating effect sizes

Type I vs Type II Error Trade-off

Balancing error types.

Inverse relationship between Type I and Type II errors
Lowering significance level (α) reduces Type I error risk but increases Type II error risk
Trade-off influenced by sample size, effect size, and research context
Researchers must consider consequences of each error type (false positives vs false negatives)
Some fields prioritize avoiding Type I errors (drug approval process)
Other areas may focus on minimizing Type II errors (environmental protection policies)

Strategies for Mitigation

Increase sample size to improve power and reduce both error types
Enhance measurement precision to reduce data variability
Consider practical significance alongside statistical significance
Use sequential testing or adaptive designs in clinical trials
Implement Bayesian approaches to incorporate prior knowledge
Conduct sensitivity analyses to assess robustness of findings
Report effect sizes and confidence intervals in addition to p-values

Key Terms to Review ( 18 )

Data Science
Data Analysis
Data Visualization
Machine Learning
Deep Learning
Computer Vision
Artificial Intelligence
AI ML DS Interview Series
AI ML DS Projects series
Data Engineering
Web Scrapping

Understanding Hypothesis Testing

Hypothesis testing involves formulating assumptions about population parameters based on sample statistics and rigorously evaluating these assumptions against empirical evidence. This article sheds light on the significance of hypothesis testing and the critical steps involved in the process.

What is Hypothesis Testing?

A hypothesis is an assumption or idea, specifically a statistical claim about an unknown population parameter. For example, a judge assumes a person is innocent and verifies this by reviewing evidence and hearing testimony before reaching a verdict.

Hypothesis testing is a statistical method that is used to make a statistical decision using experimental data. Hypothesis testing is basically an assumption that we make about a population parameter. It evaluates two mutually exclusive statements about a population to determine which statement is best supported by the sample data.

To test the validity of the claim or assumption about the population parameter:

A sample is drawn from the population and analyzed.
The results of the analysis are used to decide whether the claim is true or not.

Example: You say an average height in the class is 30 or a boy is taller than a girl. All of these is an assumption that we are assuming, and we need some statistical way to prove these. We need some mathematical conclusion whatever we are assuming is true.

Defining Hypotheses

Null hypothesis (H 0 ): In statistics, the null hypothesis is a general statement or default position that there is no relationship between two measured cases or no relationship among groups. In other words, it is a basic assumption or made based on the problem knowledge. Example : A company’s mean production is 50 units/per da H 0 : [Tex]\mu [/Tex] = 50.
Alternative hypothesis (H 1 ): The alternative hypothesis is the hypothesis used in hypothesis testing that is contrary to the null hypothesis. Example: A company’s production is not equal to 50 units/per day i.e. H 1 : [Tex]\mu [/Tex] [Tex]\ne [/Tex] 50.

Key Terms of Hypothesis Testing

Level of significance : It refers to the degree of significance in which we accept or reject the null hypothesis. 100% accuracy is not possible for accepting a hypothesis, so we, therefore, select a level of significance that is usually 5%. This is normally denoted with [Tex]\alpha[/Tex] and generally, it is 0.05 or 5%, which means your output should be 95% confident to give a similar kind of result in each sample.
P-value: The P value , or calculated probability, is the probability of finding the observed/extreme results when the null hypothesis(H0) of a study-given problem is true. If your P-value is less than the chosen significance level then you reject the null hypothesis i.e. accept that your sample claims to support the alternative hypothesis.
Test Statistic: The test statistic is a numerical value calculated from sample data during a hypothesis test, used to determine whether to reject the null hypothesis. It is compared to a critical value or p-value to make decisions about the statistical significance of the observed results.
Critical value : The critical value in statistics is a threshold or cutoff point used to determine whether to reject the null hypothesis in a hypothesis test.
Degrees of freedom: Degrees of freedom are associated with the variability or freedom one has in estimating a parameter. The degrees of freedom are related to the sample size and determine the shape.

Why do we use Hypothesis Testing?

Hypothesis testing is an important procedure in statistics. Hypothesis testing evaluates two mutually exclusive population statements to determine which statement is most supported by sample data. When we say that the findings are statistically significant, thanks to hypothesis testing.

One-Tailed and Two-Tailed Test

One tailed test focuses on one direction, either greater than or less than a specified value. We use a one-tailed test when there is a clear directional expectation based on prior knowledge or theory. The critical region is located on only one side of the distribution curve. If the sample falls into this critical region, the null hypothesis is rejected in favor of the alternative hypothesis.

One-Tailed Test

There are two types of one-tailed test:

Left-Tailed (Left-Sided) Test: The alternative hypothesis asserts that the true parameter value is less than the null hypothesis. Example: H 0 : [Tex]\mu \geq 50 [/Tex] and H 1 : [Tex]\mu < 50 [/Tex]
Right-Tailed (Right-Sided) Test : The alternative hypothesis asserts that the true parameter value is greater than the null hypothesis. Example: H 0 : [Tex]\mu \leq50 [/Tex] and H 1 : [Tex]\mu > 50 [/Tex]

Two-Tailed Test

A two-tailed test considers both directions, greater than and less than a specified value.We use a two-tailed test when there is no specific directional expectation, and want to detect any significant difference.

Example: H 0 : [Tex]\mu = [/Tex] 50 and H 1 : [Tex]\mu \neq 50 [/Tex]

To delve deeper into differences into both types of test: Refer to link

What are Type 1 and Type 2 errors in Hypothesis Testing?

In hypothesis testing, Type I and Type II errors are two possible errors that researchers can make when drawing conclusions about a population based on a sample of data. These errors are associated with the decisions made regarding the null hypothesis and the alternative hypothesis.

Type I error: When we reject the null hypothesis, although that hypothesis was true. Type I error is denoted by alpha( [Tex]\alpha [/Tex] ).
Type II errors : When we accept the null hypothesis, but it is false. Type II errors are denoted by beta( [Tex]\beta [/Tex] ).

	Null Hypothesis is True	Null Hypothesis is False
Null Hypothesis is True (Accept)	Correct Decision	Type II Error (False Negative)
Alternative Hypothesis is True (Reject)	Type I Error (False Positive)	Correct Decision

How does Hypothesis Testing work?

Step 1: define null and alternative hypothesis.

State the null hypothesis ( [Tex]H_0 [/Tex] ), representing no effect, and the alternative hypothesis ( [Tex]H_1 [/Tex] ), suggesting an effect or difference.

We first identify the problem about which we want to make an assumption keeping in mind that our assumption should be contradictory to one another, assuming Normally distributed data.

Step 2 – Choose significance level

Select a significance level ( [Tex]\alpha [/Tex] ), typically 0.05, to determine the threshold for rejecting the null hypothesis. It provides validity to our hypothesis test, ensuring that we have sufficient data to back up our claims. Usually, we determine our significance level beforehand of the test. The p-value is the criterion used to calculate our significance value.

Step 3 – Collect and Analyze data.

Gather relevant data through observation or experimentation. Analyze the data using appropriate statistical methods to obtain a test statistic.

Step 4-Calculate Test Statistic

The data for the tests are evaluated in this step we look for various scores based on the characteristics of data. The choice of the test statistic depends on the type of hypothesis test being conducted.

There are various hypothesis tests, each appropriate for various goal to calculate our test. This could be a Z-test , Chi-square , T-test , and so on.

Z-test : If population means and standard deviations are known. Z-statistic is commonly used.
t-test : If population standard deviations are unknown. and sample size is small than t-test statistic is more appropriate.
Chi-square test : Chi-square test is used for categorical data or for testing independence in contingency tables
F-test : F-test is often used in analysis of variance (ANOVA) to compare variances or test the equality of means across multiple groups.

We have a smaller dataset, So, T-test is more appropriate to test our hypothesis.

T-statistic is a measure of the difference between the means of two groups relative to the variability within each group. It is calculated as the difference between the sample means divided by the standard error of the difference. It is also known as the t-value or t-score.

Step 5 – Comparing Test Statistic:

In this stage, we decide where we should accept the null hypothesis or reject the null hypothesis. There are two ways to decide where we should accept or reject the null hypothesis.

Method A: Using Crtical values

Comparing the test statistic and tabulated critical value we have,

If Test Statistic>Critical Value: Reject the null hypothesis.
If Test Statistic≤Critical Value: Fail to reject the null hypothesis.

Note: Critical values are predetermined threshold values that are used to make a decision in hypothesis testing. To determine critical values for hypothesis testing, we typically refer to a statistical distribution table , such as the normal distribution or t-distribution tables based on.

Method B: Using P-values

We can also come to an conclusion using the p-value,

If the p-value is less than or equal to the significance level i.e. ( [Tex]p\leq\alpha [/Tex] ), you reject the null hypothesis. This indicates that the observed results are unlikely to have occurred by chance alone, providing evidence in favor of the alternative hypothesis.
If the p-value is greater than the significance level i.e. ( [Tex]p\geq \alpha[/Tex] ), you fail to reject the null hypothesis. This suggests that the observed results are consistent with what would be expected under the null hypothesis.

Note : The p-value is the probability of obtaining a test statistic as extreme as, or more extreme than, the one observed in the sample, assuming the null hypothesis is true. To determine p-value for hypothesis testing, we typically refer to a statistical distribution table , such as the normal distribution or t-distribution tables based on.

Step 7- Interpret the Results

At last, we can conclude our experiment using method A or B.

Calculating test statistic

To validate our hypothesis about a population parameter we use statistical functions . We use the z-score, p-value, and level of significance(alpha) to make evidence for our hypothesis for normally distributed data .

1. Z-statistics:

When population means and standard deviations are known.

[Tex]z = \frac{\bar{x} – \mu}{\frac{\sigma}{\sqrt{n}}}[/Tex]

[Tex]\bar{x} [/Tex] is the sample mean,
μ represents the population mean,
σ is the standard deviation
and n is the size of the sample.

2. T-Statistics

T test is used when n<30,

t-statistic calculation is given by:

[Tex]t=\frac{x̄-μ}{s/\sqrt{n}} [/Tex]

t = t-score,
x̄ = sample mean
μ = population mean,
s = standard deviation of the sample,
n = sample size

3. Chi-Square Test

Chi-Square Test for Independence categorical Data (Non-normally distributed) using:

[Tex]\chi^2 = \sum \frac{(O_{ij} – E_{ij})^2}{E_{ij}}[/Tex]

[Tex]O_{ij}[/Tex] is the observed frequency in cell [Tex]{ij} [/Tex]
i,j are the rows and columns index respectively.
[Tex]E_{ij}[/Tex] is the expected frequency in cell [Tex]{ij}[/Tex] , calculated as : [Tex]\frac{{\text{{Row total}} \times \text{{Column total}}}}{{\text{{Total observations}}}}[/Tex]

Real life Examples of Hypothesis Testing

Let’s examine hypothesis testing using two real life situations,

Case A: D oes a New Drug Affect Blood Pressure?

Imagine a pharmaceutical company has developed a new drug that they believe can effectively lower blood pressure in patients with hypertension. Before bringing the drug to market, they need to conduct a study to assess its impact on blood pressure.

Before Treatment: 120, 122, 118, 130, 125, 128, 115, 121, 123, 119
After Treatment: 115, 120, 112, 128, 122, 125, 110, 117, 119, 114

Step 1 : Define the Hypothesis

Null Hypothesis : (H 0 )The new drug has no effect on blood pressure.
Alternate Hypothesis : (H 1 )The new drug has an effect on blood pressure.

Step 2: Define the Significance level

Let’s consider the Significance level at 0.05, indicating rejection of the null hypothesis.

If the evidence suggests less than a 5% chance of observing the results due to random variation.

Step 3 : Compute the test statistic

Using paired T-test analyze the data to obtain a test statistic and a p-value.

The test statistic (e.g., T-statistic) is calculated based on the differences between blood pressure measurements before and after treatment.

t = m/(s/√n)

m = mean of the difference i.e X after, X before
s = standard deviation of the difference (d) i.e d i = X after, i − X before,
n = sample size,

then, m= -3.9, s= 1.8 and n= 10

we, calculate the , T-statistic = -9 based on the formula for paired t test

Step 4: Find the p-value

The calculated t-statistic is -9 and degrees of freedom df = 9, you can find the p-value using statistical software or a t-distribution table.

thus, p-value = 8.538051223166285e-06

Step 5: Result

If the p-value is less than or equal to 0.05, the researchers reject the null hypothesis.
If the p-value is greater than 0.05, they fail to reject the null hypothesis.

Conclusion: Since the p-value (8.538051223166285e-06) is less than the significance level (0.05), the researchers reject the null hypothesis. There is statistically significant evidence that the average blood pressure before and after treatment with the new drug is different.

Python Implementation of Case A

Let’s create hypothesis testing with python, where we are testing whether a new drug affects blood pressure. For this example, we will use a paired T-test. We’ll use the scipy.stats library for the T-test.

Scipy is a mathematical library in Python that is mostly used for mathematical equations and computations.

We will implement our first real life problem via python,

import numpy as np from scipy import stats # Data before_treatment = np . array ([ 120 , 122 , 118 , 130 , 125 , 128 , 115 , 121 , 123 , 119 ]) after_treatment = np . array ([ 115 , 120 , 112 , 128 , 122 , 125 , 110 , 117 , 119 , 114 ]) # Step 1: Null and Alternate Hypotheses # Null Hypothesis: The new drug has no effect on blood pressure. # Alternate Hypothesis: The new drug has an effect on blood pressure. null_hypothesis = "The new drug has no effect on blood pressure." alternate_hypothesis = "The new drug has an effect on blood pressure." # Step 2: Significance Level alpha = 0.05 # Step 3: Paired T-test t_statistic , p_value = stats . ttest_rel ( after_treatment , before_treatment ) # Step 4: Calculate T-statistic manually m = np . mean ( after_treatment - before_treatment ) s = np . std ( after_treatment - before_treatment , ddof = 1 ) # using ddof=1 for sample standard deviation n = len ( before_treatment ) t_statistic_manual = m / ( s / np . sqrt ( n )) # Step 5: Decision if p_value <= alpha : decision = "Reject" else : decision = "Fail to reject" # Conclusion if decision == "Reject" : conclusion = "There is statistically significant evidence that the average blood pressure before and after treatment with the new drug is different." else : conclusion = "There is insufficient evidence to claim a significant difference in average blood pressure before and after treatment with the new drug." # Display results print ( "T-statistic (from scipy):" , t_statistic ) print ( "P-value (from scipy):" , p_value ) print ( "T-statistic (calculated manually):" , t_statistic_manual ) print ( f "Decision: { decision } the null hypothesis at alpha= { alpha } ." ) print ( "Conclusion:" , conclusion )

T-statistic (from scipy): -9.0 P-value (from scipy): 8.538051223166285e-06 T-statistic (calculated manually): -9.0 Decision: Reject the null hypothesis at alpha=0.05. Conclusion: There is statistically significant evidence that the average blood pressure before and after treatment with the new drug is different.

In the above example, given the T-statistic of approximately -9 and an extremely small p-value, the results indicate a strong case to reject the null hypothesis at a significance level of 0.05.

The results suggest that the new drug, treatment, or intervention has a significant effect on lowering blood pressure.
The negative T-statistic indicates that the mean blood pressure after treatment is significantly lower than the assumed population mean before treatment.

Case B : Cholesterol level in a population

Data: A sample of 25 individuals is taken, and their cholesterol levels are measured.

Cholesterol Levels (mg/dL): 205, 198, 210, 190, 215, 205, 200, 192, 198, 205, 198, 202, 208, 200, 205, 198, 205, 210, 192, 205, 198, 205, 210, 192, 205.

Populations Mean = 200

Population Standard Deviation (σ): 5 mg/dL(given for this problem)

Step 1: Define the Hypothesis

Null Hypothesis (H 0 ): The average cholesterol level in a population is 200 mg/dL.
Alternate Hypothesis (H 1 ): The average cholesterol level in a population is different from 200 mg/dL.

As the direction of deviation is not given , we assume a two-tailed test, and based on a normal distribution table, the critical values for a significance level of 0.05 (two-tailed) can be calculated through the z-table and are approximately -1.96 and 1.96.

The test statistic is calculated by using the z formula Z = [Tex](203.8 – 200) / (5 \div \sqrt{25}) [/Tex] and we get accordingly , Z =2.039999999999992.

Step 4: Result

Since the absolute value of the test statistic (2.04) is greater than the critical value (1.96), we reject the null hypothesis. And conclude that, there is statistically significant evidence that the average cholesterol level in the population is different from 200 mg/dL

Python Implementation of Case B

import scipy.stats as stats import math import numpy as np # Given data sample_data = np . array ( [ 205 , 198 , 210 , 190 , 215 , 205 , 200 , 192 , 198 , 205 , 198 , 202 , 208 , 200 , 205 , 198 , 205 , 210 , 192 , 205 , 198 , 205 , 210 , 192 , 205 ]) population_std_dev = 5 population_mean = 200 sample_size = len ( sample_data ) # Step 1: Define the Hypotheses # Null Hypothesis (H0): The average cholesterol level in a population is 200 mg/dL. # Alternate Hypothesis (H1): The average cholesterol level in a population is different from 200 mg/dL. # Step 2: Define the Significance Level alpha = 0.05 # Two-tailed test # Critical values for a significance level of 0.05 (two-tailed) critical_value_left = stats . norm . ppf ( alpha / 2 ) critical_value_right = - critical_value_left # Step 3: Compute the test statistic sample_mean = sample_data . mean () z_score = ( sample_mean - population_mean ) / \ ( population_std_dev / math . sqrt ( sample_size )) # Step 4: Result # Check if the absolute value of the test statistic is greater than the critical values if abs ( z_score ) > max ( abs ( critical_value_left ), abs ( critical_value_right )): print ( "Reject the null hypothesis." ) print ( "There is statistically significant evidence that the average cholesterol level in the population is different from 200 mg/dL." ) else : print ( "Fail to reject the null hypothesis." ) print ( "There is not enough evidence to conclude that the average cholesterol level in the population is different from 200 mg/dL." )

Reject the null hypothesis. There is statistically significant evidence that the average cholesterol level in the population is different from 200 mg/dL.

Limitations of Hypothesis Testing

Although a useful technique, hypothesis testing does not offer a comprehensive grasp of the topic being studied. Without fully reflecting the intricacy or whole context of the phenomena, it concentrates on certain hypotheses and statistical significance.
The accuracy of hypothesis testing results is contingent on the quality of available data and the appropriateness of statistical methods used. Inaccurate data or poorly formulated hypotheses can lead to incorrect conclusions.
Relying solely on hypothesis testing may cause analysts to overlook significant patterns or relationships in the data that are not captured by the specific hypotheses being tested. This limitation underscores the importance of complimenting hypothesis testing with other analytical approaches.

Hypothesis testing stands as a cornerstone in statistical analysis, enabling data scientists to navigate uncertainties and draw credible inferences from sample data. By systematically defining null and alternative hypotheses, choosing significance levels, and leveraging statistical tests, researchers can assess the validity of their assumptions. The article also elucidates the critical distinction between Type I and Type II errors, providing a comprehensive understanding of the nuanced decision-making process inherent in hypothesis testing. The real-life example of testing a new drug’s effect on blood pressure using a paired T-test showcases the practical application of these principles, underscoring the importance of statistical rigor in data-driven decision-making.

Frequently Asked Questions (FAQs)

1. what are the 3 types of hypothesis test.

There are three types of hypothesis tests: right-tailed, left-tailed, and two-tailed. Right-tailed tests assess if a parameter is greater, left-tailed if lesser. Two-tailed tests check for non-directional differences, greater or lesser.

2.What are the 4 components of hypothesis testing?

Null Hypothesis ( [Tex]H_o [/Tex] ): No effect or difference exists. Alternative Hypothesis ( [Tex]H_1 [/Tex] ): An effect or difference exists. Significance Level ( [Tex]\alpha [/Tex] ): Risk of rejecting null hypothesis when it’s true (Type I error). Test Statistic: Numerical value representing observed evidence against null hypothesis.

3.What is hypothesis testing in ML?

Statistical method to evaluate the performance and validity of machine learning models. Tests specific hypotheses about model behavior, like whether features influence predictions or if a model generalizes well to unseen data.

4.What is the difference between Pytest and hypothesis in Python?

Pytest purposes general testing framework for Python code while Hypothesis is a Property-based testing framework for Python, focusing on generating test cases based on specified properties of the code.

Please Login to comment...

Improve your Coding Skills with Practice

What kind of Experience do you want to share?

Stack Exchange Network

Stack Exchange network consists of 183 Q&A communities including Stack Overflow , the largest, most trusted online community for developers to learn, share their knowledge, and build their careers.

Q&A for work

Connect and share knowledge within a single location that is structured and easy to search.

What is the relation of the significance level alpha to the type 1 error alpha?

In statistical hypothesis testing we decide on and set the acceptable probability of error or significance level α (alpha) to a value that fits our theory. Traditionally alpha is .1, .05, or .01.

When we calculate the power function g of the parameter we test for, we recieve the distribution of the probability of two errors: the Type 1 error α (alpha) and the Type 2 error β (beta). In a graphical representation of this function, alpha is the value below the graph, beta is the value above the line: α = g(p) and β = 1 - g(p), with p being the parameter we are interested in.

Here is an example:

The red line is α max for H 0 : p ≤ 0.4 and H 1 : p > 0.4; the blue line is β for a sample p̂ = 0.5

How do the probability of error (alpha) and the Type 1 error (alpha) relate to each other?

Or am I just getting confused over two unrelated values having the same name (alpha)?

hypothesis-testing
1 $\begingroup$ Traditionally, $\alpha = 0.05$ rather than $\alpha = 0.005$. $\endgroup$ – ocram Commented Jun 13, 2013 at 9:57
$\begingroup$ @ocram Thank you, yes, I had one 0 too many in all my "traditional alpha values". I edited my question accordingly. $\endgroup$ – user14650 Commented Jun 13, 2013 at 10:00
$\begingroup$ You seem to be talking about the same thing both times; in some circumstances, you may see people distinguish between level and significance, but in simple cases that most people encounter in practice, they refer to exactly the same thing. $\endgroup$ – Glen_b Commented Jun 13, 2013 at 10:52
$\begingroup$ How can they be the same thing? The significance level / probability of error is defined by the statistician to be a certain value, e.g. 0.05, while the probability of the Type 1 error is calculated from the values he observes and different from 0.05. $\endgroup$ – user14650 Commented Jun 13, 2013 at 12:33

2 Answers 2

When doing a continuous test and all the assumptions hold then the 2 alphas are exactly the same thing. For example if I perform a t-test on a mean and set my significance level to alpha=0.05 (or anything else) and the null hypothesis is true (the only time I can make a type I error) and all the other assumptions hold, then the probability of me making a type I error is exactly alpha.

The case where there can be a difference is when dealing with discrete probabilities. For example, I want to test if a coin is fair and plan to flip the coin 10 times. I set alpha = 0.05 as is traditional, that means that I will only reject the null hypothesis (prob=0.5) if out of 10 flips I see 0, 1, 9, or 10 heads (if I reject at 2 or 8 heads then the probability of a type I error is greater than 0.05, so would not be allowed). But if the coin is fair, then the probability of rejecting (type I error) is not 0.05, but is around 0.022 (from memory, but not that hard to compute if you want to do it yourself).

There are other cases where the true probability of a type I error may be less than the level set (one sided hypothesis is one example), so sometimes you will see alpha or the significance level defined as an upper bound on the probability of a type I error.

$\begingroup$ I understand. If I did not flip the coin n = 10 times, but n → ∞ times, the calculated true alpha would approach set alpha. Is that correct? $\endgroup$ – user14650 Commented Jun 14, 2013 at 5:55
$\begingroup$ @what, yes that is correct. $\endgroup$ – Greg Snow Commented Jun 14, 2013 at 17:09

When you are doing a statistical test, the significance level is set at the desired type I error level (alpha). So the concepts you are asking about are basically the same thing - both are fixed by design to the same value. The p-value is calculated from the data and is different from the alpha value, and may be why you are getting confused.

When doing a power calculation, typically the type I error value is fixed, as is either the available sample size, or the desired type II error level (beta). Given an expected effect size (or in the case of your graph, it appears to specify an expected proportion) the non-specified value is calculated (either necessary sample size, or available type II error level - used to get power = 1-beta).

I'm not familiar with the graph you've provided, but it appears to show how the expected effect size changes the available beta level, and demonstrate the relationship between alpha and beta. There is a natural trade-off between type I and type II error, in that if you improve one, you will worsen the other. Type I error is being calculated in this graph, but in general is not something that is calculated from your data.

Your Answer

Required, but never shown

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy .

Featured on Meta
We've made changes to our Terms of Service & Privacy Policy - July 2024
Bringing clarity to status tag usage on meta sites

Hot Network Questions

What (if any) pre-breathes were "attempted" on the ISS, and why?
Passive Construction - Standard Form vs. Non-Standard Form
How much effort to repair front porch footings/girders?
Can you successfully substitute pickled onions for baby onions in Coq Au Vin?
Can I repair these deck support posts, or do I need to replace them?
Reed-Solomon error count if errors cannot be corrected
What Christian ideas are found in the New Testament that are not found in the Old Testament?
Can the speed of light inhibit the synchronisation of a power grid?
Book about a colony ship making an unscheduled stop in a star system with no habitable planets
If you get pulled for secondary inspection at immigration, missing flight, will the airline rebook you?
Is the Shroud of Turin about 2000 years old?
1970s? Novel, a man is stuck/trapped? in a city where the other people are fooled/conned into believing things are better than they are
Everyone hates this Key Account Manager, but company won’t act
In TNG: the Pegasus, why is Geordi in the first meeting with the Admiral?
What sort of impact did the discovery that water could be broken down (via electrolysis) into gas have?
Home water pressure higher than city water pressure?
Chord definition misunderstanding
Why are swimming goggles typically made from a different material than diving masks?
Does gluing two points prevent simple connectedness?
Should I Inform My PhD Program About Not Completing My Master's Degree Due to Personal Challenges?
Random Oracle in Fiat-Shamir Transform (from Katz-Lindell Textbook)
Who said "If you don't do politics, politics will do you"?
Reference request: "Higher order eigentuples" as generalized eigenvectors?
How does a closed-cycle rocket engine keep the chamber pressure from stalling the pump turbine?

IMAGES

An easy-to-understand summary of significance level
Significance Level and Power of a Hypothesis Test Tutorial
Significance Level and Power of a Hypothesis Test Tutorial
Understanding Hypothesis Tests: Significance Levels (Alpha) and P
Significance Level
PPT

COMMENTS

Understanding Hypothesis Tests: Significance Levels (Alpha) and P
The P value of 0.03112 is statistically significant at an alpha level of 0.05, but not at the 0.01 level. If we stick to a significance level of 0.05, we can conclude that the average energy cost for the population is greater than 260. A common mistake is to interpret the P-value as the probability that the null hypothesis is true.
How Hypothesis Tests Work: Significance Levels (Alpha) and P values
Using P values and Significance Levels Together. If your P value is less than or equal to your alpha level, reject the null hypothesis. The P value results are consistent with our graphical representation. The P value of 0.03112 is significant at the alpha level of 0.05 but not 0.01.
Understanding Significance Levels in Statistics
While this post looks at significance levels from a conceptual standpoint, learn about the significance level and p-values using a graphical representation of how hypothesis tests work. Additionally, my post about the types of errors in hypothesis testing takes a deeper look at both Type 1 and Type II errors, and the tradeoffs between them.
An Easy Introduction to Statistical Significance (With Examples)
The p value determines statistical significance. An extremely low p value indicates high statistical significance, while a high p value means low or no statistical significance. Example: Hypothesis testing. To test your hypothesis, you first collect data from two groups. The experimental group actively smiles, while the control group does not.
Hypothesis Testing, P Values, Confidence Intervals, and Significance
Medical providers often rely on evidence-based medicine to guide decision-making in practice. Often a research hypothesis is tested with results provided, typically with p values, confidence intervals, or both. Additionally, statistical or research significance is estimated or determined by the investigators. Unfortunately, healthcare providers may have different comfort levels in interpreting ...
Hypothesis Testing
Let's return finally to the question of whether we reject or fail to reject the null hypothesis. If our statistical analysis shows that the significance level is below the cut-off value we have set (e.g., either 0.05 or 0.01), we reject the null hypothesis and accept the alternative hypothesis. Alternatively, if the significance level is above ...
Hypothesis Testing
Hypothesis testing is a formal procedure for investigating our ideas about the world using statistics. It is most often used by scientists to test specific predictions, called hypotheses, that arise from theories. ... And in most cases, your predetermined level of significance for rejecting the null hypothesis will be 0.05 ...
7.5: Critical values, p-values, and significance level
When we use z z -scores in this way, the obtained value of z z (sometimes called z z -obtained) is something known as a test statistic, which is simply an inferential statistic used to test a null hypothesis. The formula for our z z -statistic has not changed: z = X¯¯¯¯ − μ σ¯/ n−−√ (7.5.1) (7.5.1) z = X ¯ − μ σ ¯ / n.
5.1
A test is considered to be statistically significant when the p-value is less than or equal to the level of significance, also known as the alpha ( α) level. For this class, unless otherwise specified, α = 0.05; this is the most frequently used alpha level in many fields. Sample statistics vary from the population parameter randomly.
Hypothesis Testing
Perform a hypothesis test, with significance level 0.05, to test if there is enough evidence to conclude that the population mean is not equal to 200 mg/dL. Hypothesis Test. We will perform a hypothesis test using the $p$-value approach with significance level $\alpha=0.05$ and the $t$-distribution with 24 degrees of freedom: ...
Level of Significance & Hypothesis Testing
The level of significance is defined as the criteria or threshold value based on which one can reject the null hypothesis or fail to reject the null hypothesis. The level of significance determines whether the outcome of hypothesis testing is statistically significant or otherwise. The significance level is also called as alpha level.
Hypothesis Testing and Confidence Intervals
The relationship between the confidence level and the significance level for a hypothesis test is as follows: Confidence level = 1 - Significance level (alpha) For example, if your significance level is 0.05, the equivalent confidence level is 95%. Both of the following conditions represent statistically significant results: The P-value in a ...
3.1: The Fundamentals of Hypothesis Testing
Given sample data, a level of significance of 5%, and an alternative weight of 9.2 lb., we can compute the power of the test to reject μ = 8.7 lb. If we have a small sample size, the power will be low. However, increasing the sample size will increase the power of the test. Increasing the level of significance will also increase power.
Significance Level and Power of a Hypothesis Test
1. Significance Level. Before we begin, you should first understand what is meant by statistical significance. When you calculate a test statistic in a hypothesis test, you can calculate the p-value. The p-value is the probability that you would have obtained a statistic as large (or small, or extreme) as the one you got if the null hypothesis ...
Understanding P-Values and Statistical Significance
In statistical hypothesis testing, you reject the null hypothesis when the p-value is less than or equal to the significance level (α) you set before conducting your test. The significance level is the probability of rejecting the null hypothesis when it is true. Commonly used significance levels are 0.01, 0.05, and 0.10.
Hypothesis Testing: Significance Level & Rejection Region
So, we can choose a higher significance level like 0.05 or 0.1. Hypothesis Testing: Performing a Z-Test. Now that we have an idea about the significance level, let's get to the mechanics of hypothesis testing. Imagine you are consulting a university and want to carry out an analysis on how students are performing on average.
Hypothesis Testing: Upper-, Lower, and Two Tailed Tests
We will assume the sample data are as follows: n=100, =197.1 and s=25.6. Step 1. Set up hypotheses and determine level of significance. H 0: μ = 191 H 1: μ > 191 α =0.05. The research hypothesis is that weights have increased, and therefore an upper tailed test is used. Step 2.
Level of Significance (Statistical Significance)
The level of significance is defined as the fixed probability of wrong elimination of null hypothesis when in fact, it is true. ... This process is termed as statistical hypothesis testing. The level of significance or Statistical significance is an important terminology that is quite commonly used in Statistics. In this article, we are going ...
T-test and Hypothesis Testing (Explained Simply)
The significance level is the desired probability of rejecting the null hypothesis when it is true. For instance, if a researcher selects α=0.05, it means that he is willing to take a 5% risk of falsely rejecting the null hypothesis.
Tests of Significance
Significance Levels The significance level for a given hypothesis test is a value for which a P-value less than or equal to is considered statistically significant. Typical values for are 0.1, 0.05, and 0.01. These values correspond to the probability of observing such an extreme value by chance. In the test score example above, the P-value is 0.0082, so the probability of observing such a ...
S.3.2 Hypothesis Testing (P-Value Approach)
The P -value is, therefore, the area under a tn - 1 = t14 curve to the left of -2.5 and to the right of 2.5. It can be shown using statistical software that the P -value is 0.0127 + 0.0127, or 0.0254. The graph depicts this visually. Note that the P -value for a two-tailed test is always two times the P -value for either of the one-tailed tests.
6.2 Type I and Type II errors, significance level, and power
Significance Level: The significance level is a threshold in statistical hypothesis testing that determines when to reject the null hypothesis. It is commonly denoted by the symbol $$\alpha$$ and represents the probability of making a Type I error, which occurs when the null hypothesis is incorrectly rejected when it is actually true.
Understanding Hypothesis Testing
If your P-value is less than the chosen significance level then you reject the null hypothesis i.e. accept that your sample claims to support the alternative hypothesis. Test Statistic: The test statistic is a numerical value calculated from sample data during a hypothesis test, used to determine whether to reject the null hypothesis. It is ...
hypothesis testing
In statistical hypothesis testing we decide on and set the acceptable probability of error or significance level α (alpha) to a value that fits our theory ...

Understanding Hypothesis Tests: Significance Levels (Alpha) and P values in Statistics

What Is the Significance Level (Alpha)?

Ready for a demo of Minitab Statistical Software? Just ask!

What Are P values?

Discussion about Statistically Significant Results

You Might Also Like

StatPearls [Internet].

Affiliations

In this Page

Related information

Similar articles in PubMed

Recent Activity

Hypothesis Testing (cont...)

Significance levels

One- and two-tailed predictions

Rejecting or failing to reject the null hypothesis

Have a language expert improve your writing

Hypothesis Testing | A Step-by-Step Guide with Easy Examples

Table of contents

Here's why students love Scribbr's proofreading services

Cite this Scribbr article

Is this article helpful?

Rebecca Bevans

Hypothesis Testing

Definitions and Methodology

Level of Significance & Hypothesis Testing

What is Hypothesis testing and how is it related to significance level?

What is the level of significance?

Why does one need a level of significance?

How is the level of significance used in hypothesis testing?

Level of Significance – Quiz / Interview Questions

#1. The p-value less than the level of significance would mean which of the following?

Ajitesh Kumar

Leave a Reply Cancel reply

ChatGPT Prompts (250+)

Data Science / AI Trends

Free Online Tools

Recent Comments

Hypothesis Testing and Confidence Intervals

Significance Level vs. Confidence Level

Confidence Intervals and the Inherent Uncertainty of Using Sample Data

Confidence Intervals and Statistical Significance

Hypothesis Testing and Confidence Intervals Always Agree

I Really Like Confidence Intervals!

Share this:

Reader Interactions

Comments and Questions Cancel reply

First, please create an account

1. Significance Level

2. Power of a Hypothesis Test

P-Value And Statistical Significance: What It Is & Why It Matters

Hypothesis testing

What a p-value tells you

Example: Test Statistic and p-Value

P-value interpretation

A p-value less than or equal to your significance level (typically ≤ 0.05) is statistically significant.

Example: Statistical Significance

What does a p-value of 0.001 mean?

A p-value more than the significance level (typically p > 0.05) is not statistically significant and indicates strong evidence for the null hypothesis.

One-Tailed Test

Two-Tailed Test

How do you calculate the p-value ?

Example: Choosing a Statistical Test

How to report

Example: Reporting the results

Why is the p -value not enough?

When do you reject the null hypothesis?

What does p-value of 0.05 mean?

Are all p-values below 0.05 considered statistically significant?

How does sample size affect the interpretation of p-values?

Can a non-significant p-value indicate that there is no effect or difference in the data?

Can P values be exactly zero?

Further Information

Hypothesis Testing with Z-Test: Significance Level and Rejection Region

What Is the Significance Level?

How Is the Significance Level Denoted?

A Case in Point

Higher Degree of Error

Hypothesis Testing: Performing a Z-Test

Visualizing the Grades